stress strain curve research papers

Stress Strain Curves

• Open Access
• First Online: 10 January 2024

Cite this chapter

You have full access to this open access chapter

• Rolf Sandström 12  

Part of the book series: Springer Series in Materials Science ((SSMATERIALS,volume 339))

2548 Accesses

Traditionally, stress strain curves for example from tensile testing are described with empirical models with a number of adjustable parameters such as Hollomon, Ludwik, Voce and Swift. With such models it is difficult or impossible to generalize and extrapolate. A model in the form of Voce equation is derived from the same basic dislocation model used for the creep models with the values of constants computed. The derived model is used to describe stress strain curves for Cu including their temperature and strain rate dependence. The dynamic recovery constant ω plays a central to show how the work hardening deviates from a linear behaviour. The temperature dependence of ω is analyzed and shown to be related to that of the shear modulus. In the literature it is frequently assumed that dynamic recovery is controlled by cross-slip. However, the measured activation energy for dynamic recovery is many times smaller than the energy required to make partial dislocations brought together and form a constriction, which is necessary to enable cross-slip, so this is an unlikely possibility.

You have full access to this open access chapter,  Download chapter PDF

3.1 General

Stress strain curves are usually generated with the help of tensile tests. In a tensile test a specimen is exposed to a constant length expansion rate at the same time as the force is recorded. The expansion rate is transferred to strain by dividing it by the initial specimen gauge length. The stress is obtained by dividing the force by the initial specimen cross section. In this way a stress versus strain curve is generated for the material, which is also referred to as a flow curve.

Tensile tests are performed on a large scale since the test is used to check the properties of batches during metal production. From the stress strain curves the strength and ductility of the material can be determined. The strength that is a measure of the initiation of plastic deformation, the yield strength, is taken at the linear offset by 0.2% strain for most material. The maximum load in the flow curve gives the maximum stress a material can take, the tensile strength. It is often thought that the strength is the most important property of a material. However, that is not necessarily the case. In fact, in order to allow for a more flexible use of a material, it must have a certain amount of formability. In a tensile test, the formability can be measured as the (total) elongation, which is determined as the strain at failure. In scientific work, also the uniform elongation is used, which is the strain at the maximum load in the tensile test. The elongation is also referred to as the ductility that can be said to be another word for formability. Elongation is not the only way to measure the ductility in a tensile test. Another way is to determine the reduction in the specimen cross section after failure, reduction of area.

The ductility is quite an important property. For example, a low ductility makes the material sensitive to overloads, fatigue loads and scratches and other marks on the surface. This can be exemplified for many ceramic materials that have virtually nil ductility. Special design procedures are required to avoid premature failures with low ductility. For example, tensile stresses, sharp corners and rough surfaces must be avoided.

In materials production, stress strain curves from tensile tests are recorded but not usually modeled. If they are, empirical methods are used. Examples of empirical methods for analyzing stress strain curves will be given in Sect.  3.2 . In the same way as for creep tests, basic modeling is needed to safely determine the operating mechanisms. This applies also to flow curves from tensile tests. Unfortunately, scientific efforts to formulate such models have been quite limited. However, some models will be presented in Sect.  3.3 . The results from tensile tests and creep tests can be assumed to be controlled by the same plastic deformation mechanisms. Thus, the data from tensile tests can significantly supplement the information recorded from creep tests. The dynamic recovery parameter ω plays an important role for the work hardening behavior. In particular, it describes how fast the deviation from a linear stress strain curve takes place. Its value and temperature dependence are discussed in Sect.  3.4 .

3.2 Empirical Methods to Describe Stress Strain Curves

To describe stress strain curves, there are many empirical methods in the literature. Some of the classical approaches are listed in Eqs. ( 3.1 – 3.4 ). Ludwik’s equation is by far the oldest, but the other three have been around since about 1950. References to the original papers can be found in [ 1 ]

σ is the stress and ε is the strain. The other quantities are adjustable parameters that are fitted to the data. The use of the equations will be illustrated for copper and for a stainless steel. In Fig.  3.1 data for phosphorus alloyed pure copper (Cu-OFP) is shown in two conditions and compared with fitted curves using Eqs. ( 3.1 )–( 3.4 ).

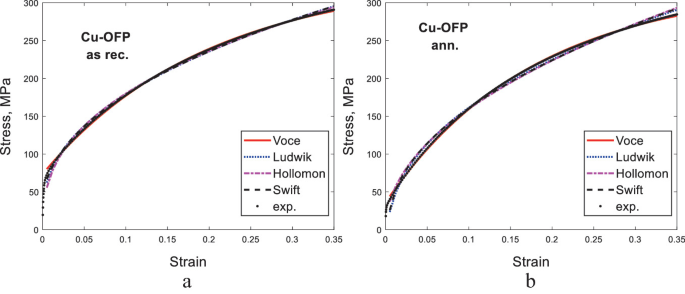
Stress versus strain for Cu-OFP at room temperature; comparison between four empirical models and experimental data; a as received condition (forged); b annealed condition (450 °C, 3 h) (unpublished data)

The two conditions give quite similar curves. However, there are some small differences. In the annealed condition the data have a slightly larger curvature and the yield strength is lower in comparison to the as received condition. The reason is that a small amount of cold working remains after the forging in the as received state.

In general an acceptable fit to the data is obtained. There are some slight deviations though. The Ludwik and the Swift equations do not fit the data fully in the annealed condition. At small strains below 0.02 there are significant deviations for both conditions. For the as received condition the Ludwik and the Swift equations seem to work best whereas the situation is the opposite for the other condition where the Voce and Hollomon methods seem to be somewhat better.

The situation at small strains can readily be improved by extending the Eqs. ( 3.1 )–( 3.4 ) somewhat, A direct way is to combine the Ludwik and Voce expressions, Eq. ( 3.5 )

Another way is to duplicate the fitting terms. This is illustrated in Eqs. ( 3.6 ) and ( 3.7 )

In the literature Ludwigson’s Eq. ( 3.8 ) has been cited many times [ 2 ]. It is the same as Eq. ( 3.5 ) but without the constant term. Unfortunately, this turns it out to make it more difficult to use the equation.

The application of Eqs. ( 3.5 )–( 3.7 ) to copper is illustrated in Figs.  3.2 and 3.3 .

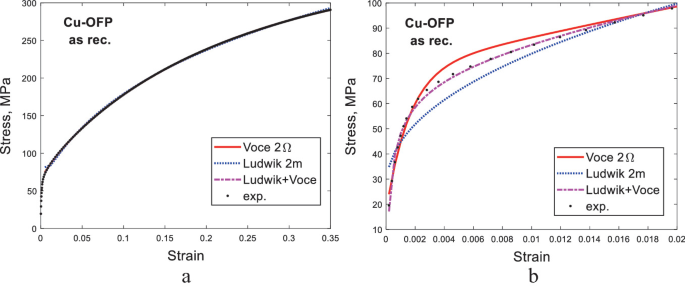
Stress versus strain for Cu-OFP at room temperature in as received condition; a fit to Eqs. ( 3.5 )–( 3.7 ); b enlargement of the low strain part of ( a )

Stress versus strain for Cu-OFP at room temperature in the annealed condition; a fit to Eqs. ( 3.5 )–( 3.7 ); b enlargement of the low strain part of ( a )

It can be seen that with the extended Eqs. ( 3.5 )–( 3.7 ) the fit at low strains can be much improved. To see any deviation one has to go to the enlargements in Figs.  3.2 and 3.3 . There it can be seen that the best result is obtained for the Voce + Ludwik method in the as-received condition and the Voce 2Ω method in the annealed condition.

In Fig.  3.4 , data for the austenitic stainless steel 17Cr12Ni2Mo0.08C (316H) at room temperature and 800 °C are illustrated.

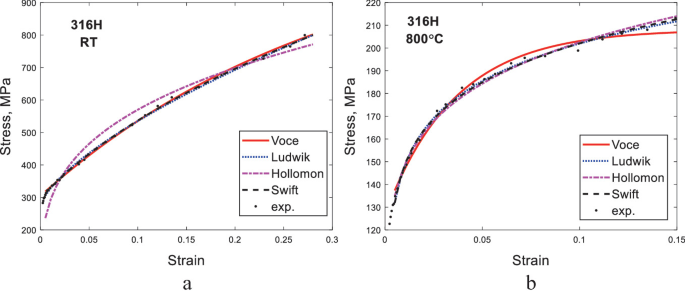
Stress versus strain for the austenitic stainless steel 17Cr12Ni2Mo0.08C (316H); a at room temperature; b at 800 °C. Equations ( 3.1 )–( 3.4 ) are compared to experimental data from [ 1 ]

From Fig.  3.4 a it is evident that the Hollomon equation does not work very well in this case. At 800 °C, Eqs. ( 3.1 ), ( 3.2 ) and ( 3.4 ) are satisfactory but not the Voce equation. Handling of low strains is less problematic than for the copper alloys. However, if a higher precision is needed the extended equations can be applied for the stainless steels as well. This is shown in Figs.  3.5 and 3.6 .

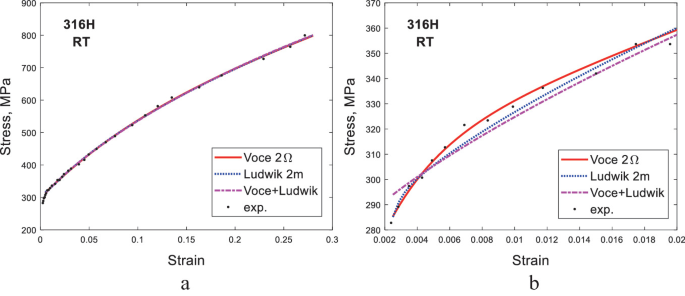
Comparison to the extended Eqs. ( 3.5 )–( 3.7 ) for the same data for 316H at room temperature as in Fig.  3.4 a; a full curve; b enlargement of the low strain part of ( a )

Comparison to the extended Eqs. ( 3.5 )–( 3.7 ) for the same data for 316H at 800 °C as in Fig.  3.4 b; a full curve; b enlargement of the low strain part of ( a )

It is evident from Figs.  3.5 a and 3.6 a, the three extended methods work well for the stainless steel both at room temperature and at 800 °C: In Fig.  3.5 b, the Voce 2Ω method seems to be the best alternative. At the high temperature all the extended methods are satisfactory also at low strains, Fig.  3.6 .

There is a general experience that it is not simple to decide in advance which empirical relation would give the best fit. This has also been illustrated by the examples above. Most of Eqs. ( 3.1 )–( 3.7 ) are purely empirical and cannot be derived from basic physical principles. The exception is the Voce equation. This will be demonstrated in detail in the next section. The other methods are purely empirical. This means that they are flexible expressions that are suitable for data fitting. One of the risks with empirical expressions is that when you get a good fit to the data it is tempting to draw the conclusion that is has physical significance, but that should clearly be avoided.

In Eqs. ( 3.1 )–( 3.8 ) above 2–5 adjustable parameters are present that are to be fitted to the experimental data. There are many types of software that can handle that task. However, it has been found that the fitting process can be slow in particular when the number of adjustable parameters is large. In addition one cannot automatically be sure that the parameters converge to the desired result. The situation is different if there is only a linear dependence of the parameter. An example of that is the determination of constants when a time temperature parameter is used to extrapolate creep rupture data. The problem can be formulated in such a way that the function to be fitted is linear in the adjustable parameters. In such a case the parameter values can be found by solving a linear equation, which is instant. Equations ( 3.1 )–( 3.8 ) are linear in a 1 , a 2 and a 3 , whereas m 1 , m 2 , Ω 1 and Ω 2 are nonlinear parameters. By fitting the nonlinear parameters by the optimization software and the linear parameters by solving a linear equation, the accuracy and efficiency of the process can be much improved.

3.3 Basic Model

3.3.1 the model.

Tensile tests are performed at a constant expansion rate or approximately constant strain rate. Creep strain and rupture tests are carried out at constant load or stress. In spite of the difference in testing conditions it is assumed that the same mechanisms are controlling the plastic deformation in both cases. This means that the equations developed in Chap. 2 should be valid also for stress strain curves. The basic expression for the development of the dislocation density ρ is given by Eq. ( 2.17 )

where ε is the strain and \({\dot{\upvarepsilon }}\) the strain rate, m T the Taylor factor, b burgers vector, c L a dimensionless factor, ω the dynamic recovery factor, τ L the dislocation line tension, and M cl the dislocation climb mobility. These quantities are further explained in Sect.  2.3 . The three terms on the right hand side (RHS) of Eq. ( 3.9 ) represent the contributions from work hardening, dynamic recovery and static recovery. At low strains the work hardening term dominates. Thus at this stage the dislocation density ρ increases with increasing strain. Due to different dependence on ρ of the three terms in Eq. ( 3.9 ), the dynamic recovery term increases faster with strain than the work hardening term, and the static recovery term faster than the dynamic recovery term. This means that the rate of increase in ρ drops with increasing strain. Eventually the recovery terms are so large that there is a balance between these terms and the work hardening. This balance cannot always be observed in a tensile test, because an instability might occur before the balance is reached and the specimen fails. This instability will be discussed below.

For stress strain curves, the last term in Eq. ( 3.9 ), the static recovery term is often small and can be neglected. The role of this term will still be analyzed below. If the static recovery term is ignored, Eq. ( 3.9 ) can be integrated directly

We find as expected that ρ increases with strain and reaches saturation value at large strains. The Taylor equation is used to transfer the dislocation density to strength

where σ disl is the strength contribution from the dislocations, σ y the yield strength, α ≈ 0.19 a constant, and G the shear modulus. If Eq. ( 3.10 ) is inserted into Eq. ( 3.11 ), the strain dependence of the strength is obtained

It is convenient to introduce the saturation stress σ sat

By introducing the saturation stress, Eq. ( 3.12 ) can be rewritten as

In Eq. ( 3.14 ), the yield strength depends on the temperature and the strain rate. A model for this dependence is presented in [ 3 ].

The yield strength is first given at a reference temperature \( T_{0}\) and reference strain rate \( {\dot{\upvarepsilon }}_{0}\) that are usually taken as 20 °C and 1 × 10 –4 1/s, respectively. The change in the yield strength with temperature is assumed to follow that of the shear modulus G . The strain rate dependence is described with a Norton equation. The stress exponent n N is determined at the temperature and strain rate in question from the equation for the secondary creep rate, Eq. ( 2.30 ). The shear modulus G ( T ) is assumed to be approximately linear in temperature. Equations ( 3.12 ) and ( 3.14 ) have exactly the form of the Voce Eq. ( 3.3 ) with

Equation ( 3.9 ) has been used for many years to derive the Voce equation, see for example [ 4 , 5 , 6 ]. In these papers, a number of the constants were used as adjustable parameters. This is no longer necessary. All the constants in Eq. ( 3.16 ) are given except σ y that is taken as an experimental value. σ y varies significantly for different material batches and cannot be accurately predicted at present. But the full strain dependence can be described. The constant α requires a comment. The contribution to α can be split into a short range part α S and a long range part α G [ 7 , 8 ]. The long range part has been explicitly derived [ 8 ]

where ν P is Poisson’s ratio. With ν P  = 0.3, α G  = 0.19. At elevated temperatures, α ≈ α G [ 7 ]. As a consequence, α = 0.19 is used in this book.

At the end of Sect.  2.4 , a special procedure was described for finding the value of the work hardening parameter c L based on the assumption that dynamic and static recovery give the same result. An expression for the maximum dislocation density ρ s in this situation was given in Eq. ( 2.26 ). This is equation is now expressed in stress instead of the dislocation density with the help of the Taylor Eq. ( 3.11 )

τ L is dislocation line tension, M cl the climb mobility and σ i the contribution from particle and solid solution hardening including the yield strength. In general, Eq. ( 3.18 ) has to be iterated to find the value of the saturation stress, but the iteration usually converges quickly. With Eq. ( 3.13 ), the value of c L is obtained

A plastic instability in a tensile test takes place when Considère’s criterion is satisfied

Applying Considère’s criterion to Eq. ( 3.14 ), the maximum stress σ max in a tensile test can be obtained as well as the strain at this position, which is referred to as the uniform elongation ε u .

With ω ≈ 15 for copper and some stainless steels [ 1 ], the maximum stress σ max is about 10% less than the saturation stress σ sat . The uniform elongation ε u is often measured. ε u is an important property because it is much easier to predict than the total elongation where the role of specimen necking must be taken into account. This will be considered in Chap. 12 for tertiary creep.

3.3.2 Application to Parent Metal

In Fig.  3.7 tensile data for Cu-OFP at 75 °C are presented. A comparison is made to the model that is obtained by integrating Eq. ( 3.9 ) and combining it with the Taylor equation, Eq. ( 3.11 ). To investigate the significance of the static recovery term (the last term in Eq. ( 3.9 )), results are shown both with and without this contribution. It is evident that static recovery has a negligible influence in this case.

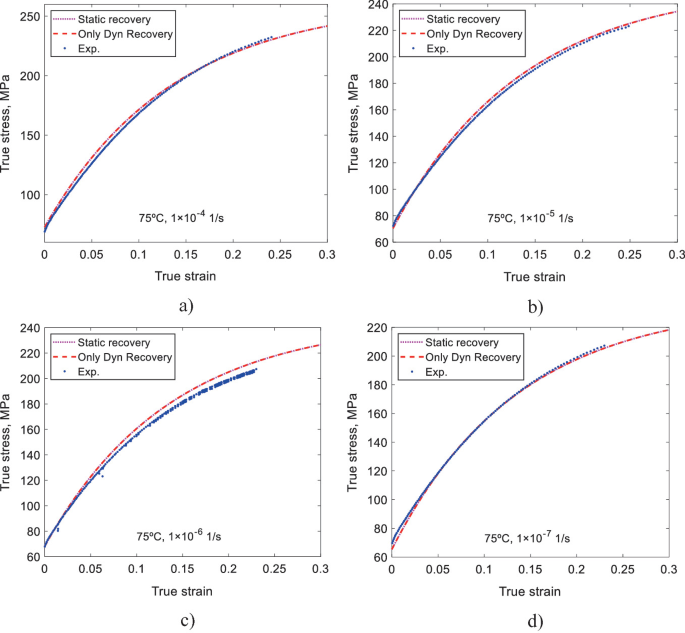
Stress versus strain for Cu-OFP at 75 °C comparing the model in Eqs. ( 3.9 ) and ( 3.11 ) with experiments. Data from [ 3 ]. To analyze the role of static recovery (last term in Eq. ( 3.9 )), results with and without this term are shown; a 1 × 10 –4 1/s; b 1 × 10 –5 1/s; c 1 × 10 –6 1/s; d 1 × 10 –7 1/s

In Fig.  3.8 results at 125 °C are given. The model results in this case involve all the terms in Eq. ( 3.9 ) including the static recovery term.

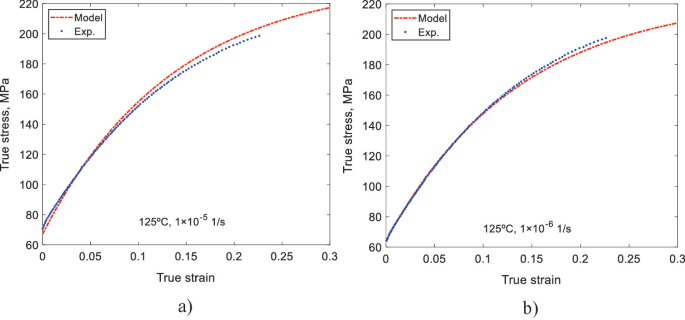
Stress versus strain for Cu-OFP at 125 °C comparing the model in Eqs. ( 3.9 ) and ( 3.11 ) with experiments. Data from [ 3 ]; a 1 × 10 –5 1/s; b 1 × 10 –6 1/s

In Fig.  3.9 experimental and model results for Cu-OFP at 175 °C are given. Only predicted values for the parameters in Eqs. ( 3.9 ) and ( 3.11 ) are used. The exception is the yield strength. Its value at 75 °C and 1 × 10 –4 1/s is taken from experiments. The yield strength value at other conditions are derived using the Eq. ( 3.15 ).

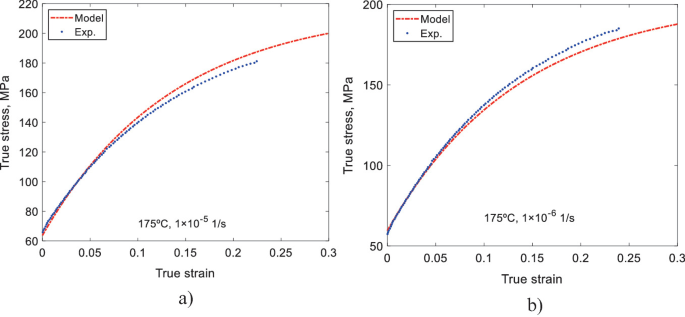
Stress versus strain for Cu-OFP at 175 °C comparing the model in Eqs. ( 3.9 ) and ( 3.11 ) with experiments. Data from [ 3 ]; a 1 × 10 –5 1/s; b 1 × 10 –6 1/s

3.3.3 Application to Welds

Equations ( 3.9 ) and ( 3.11 ) have also been applied to friction stir welds in phosphorus alloyed oxygen copper Cu-OFP. To describe welds is always challenging since the properties can locally vary significantly. The same parameters as for the parent metal have been used except for ω (= 12) and the yield strength where one value has been taken from the experiments at 75 °C and 1 × 10 –4 . Why the ω value had to be reduced is not understood. In Fig.  3.10 examples are given for the weld zone.

Stress versus strain for the weld zone in friction stir welds in Cu-OFP comparing the model in Eqs. ( 3.9 ) and ( 3.11 ) with experiments. Data from [ 9 ]; a 20 °C, 1 × 10 –4 1/s; b 75 °C, 3 × 10 –6 1/s

The heat affected zone (HAZ) has also been studied. Examples are presented in Fig.  3.11 . The behavior of the whole weld (the cross weld) usually has to be derived from the properties of the weld zone and the HAZ. However, since the properties of the weld are essentially the same and close to those of the parent metal, it is reasonable to think that the weld can be handled in the same way and that has been assumed. Examples for cross welds are shown in Fig.  3.12 .

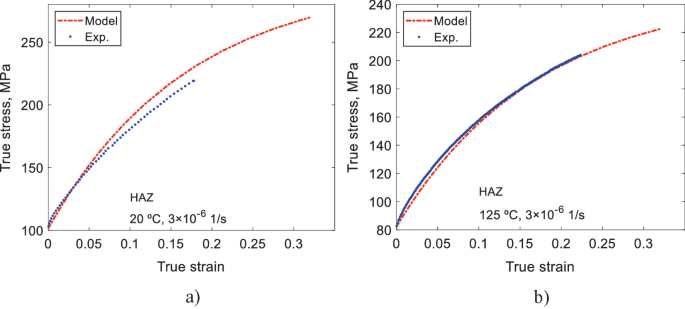
Stress versus strain for the heat affected zone (HAZ) in friction stir welds in Cu-OFP at 75 °C comparing the model in eqs. ( 3.9 ) and ( 3.11 ) with experiments. Data from [ 9 ]; a 20 °C, 3 × 10 –6 1/s; b 125 °C, 3 × 10 –6 1/s

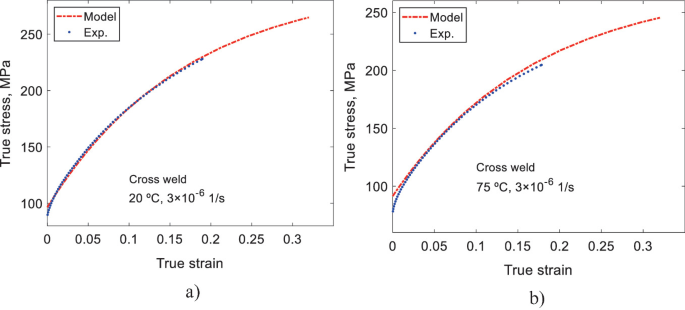
Stress versus strain for cross welds in friction stir welds in Cu-OFP comparing the model in Eqs. ( 3.9 ) and ( 3.11 ) with experiments. Data from [ 9 ]; a 20 °C, 3 × 10 –6 1/s; b 75 °C, 3 × 10 –6 1/s

It can be concluded that the dislocation model in ( 3.9 ) and ( 3.11 ) can represent stress strain curves for both parent metals and welds of Cu-OFP in quite a reasonable way essentially without the use of adjustable parameters. The analysis above is likely to be valid for other fcc alloys as well. However, some parameters and in particular ω will take other values. This will analyzed in the next section.

3.4 The ω Parameter in Dynamic Recovery

As was emphasized in Sect.  2.3 , two types of recovery (dynamic and static) are important to take into account when discussing creep. It is also important to distinguish between the two types of recovery. The primary role of dynamic recovery is to provide the reduction of the work hardening rate with increasing strain in stress strain curves. Static recovery reduces the dislocation content during creep, which makes it possible for creep deformation to continue at constant load or stress. Static recovery must be considered to be well understood. At high temperatures static recovery is assumed to control the creep rate and the temperature and stress dependence of the creep rate has been studied for many alloys. In this way detailed knowledge about static recovery has been gathered indirectly. The models for static recovery are based on expressions for a basic model of the climb mobility, which was established many years ago.

Unfortunately, the modeling of dynamic recovery is not at all at the same level. This is surprising since it has been seen earlier in this chapter that dynamic recovery is an essential part of the description of plastic flow curves at ambient and intermediate temperatures. As can be seen for example in Fig.  3.7 , the slope of the curve which is referred to as the work hardening rate is continuously decreasing with increasing strain that is the effect of dynamic recovery. This slope can directly be obtained from Eq. ( 3.12 ) if the Voce equation is satisfied

The initial work hardening rate which is the constant in front of the exponential is found to take a value of about G /20 for polycrystalline fcc alloys [ 4 ]. The work hardening rate in Eq. ( 3.23 ) can also be expressed in terms of the stress. By using Eqs. ( 3.12 ) and ( 3.13 ), Eq. ( 3.23 ) can be transformed to

This linear behavior of the work hardening rate as a function of stress has been demonstrated in many papers, see for example [ 10 ].

According to Eq. ( 3.9 ), the amount of dynamic recovery is directly proportional to the parameter ω. Consequently, the value of ω is of main importance for understanding dynamic recovery. For example, it is evident from Eqs. ( 3.23 ) and ( 3.24 ) that ω has major influence on the work hardening rate. Unfortunately, only limited data for ω is available in the literature. Data for austenitic stainless steels are shown in Fig.  3.13 .

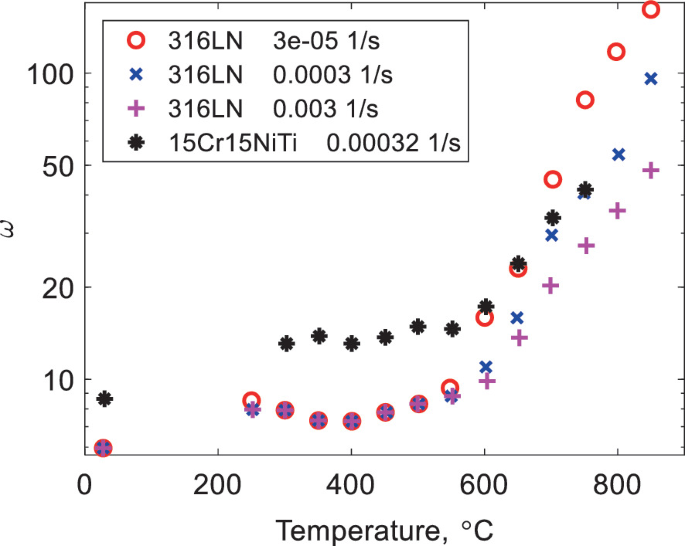
The dynamic recovery parameter ω as a function of temperature for two austenitic stainless steels. Data from [ 1 , 11 ]

Different designations for ω can be found in the literature. For stress strain curves Ω and n V are often used. They are related to ω in the following way

It is not uncommon that values for ω and Ω are mixed up so it is wise to check the data.

It is evident from Fig.  3.13 that one should distinguish between two ranges for the temperature dependence of ω. At high temperatures in the creep range, the ω values are strongly dependent on both temperature and strain rate. For austenitic stainless steels, the creep range starts at about 550 °C. At lower temperatures, ω is weakly temperature dependent and the strain rate has quite a small effect. This is called the work hardening range, because the flow curves are primarily controlled by the work hardening rate. This behavior has also been found for aluminum [ 12 ].

When mechanisms are considered the work hardening and creep range are defined in the following way

σ sat is the maximum flow stress in a stress strain curve. For a material satisfying the Voce equation, σ sat is given by Eq. ( 3.13 ). σ creep is the stress in a creep test that gives the same strain rate as for the stress strain flow curve. This division is illustrated in Fig.  3.14 .

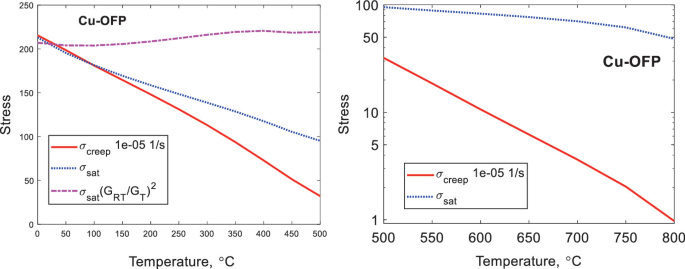
Saturation and creep stress as a function of temperature for Cu-OFP at a strain rate of 1 × 10 –5 1/s. A third curve gives the saturation stress multiplied by \((G_{RT} /G_{T} )^{2}\) where G RT and G T are the shear modulus at room temperature and temperature, respectively

Below about 100 °C, \(\upsigma _{{{\text{sat}}}} <\upsigma _{{{\text{creep}}}}\) and we are in the work hardening range in Fig.  3.14 . The situation is reversed above this temperature. Below 400 °C ( T m /2 for Cu, T m the absolute melting point) both σ sat and σ creep decrease linearly with increasing temperature but with different slope. Above 400 °C, σ creep decreases exponentially with increasing temperature, a behavior that is frequently observed for creep resistant steels. It is natural to distinguish between three temperature ranges: the high temperature range ( T  >  T m /2), the intermediate range (0.3 T m  <  T  <  T m /2, 130–400 °C for Cu) and the low temperature range ( T  < 0.3 T m ). The high temperature range is the classical region for studying creep. Dynamic recovery is the dominating recovery process only in the low temperature range.

There is not a sharp transition between the low and intermediate temperature ranges. In fact, we can have \(\upsigma _{{{\text{sat}}}} >\upsigma _{{{\text{creep}}}}\) in spite σ creep being above the experimental data range in a flow curve in the lower part of the intermediate range. In Fig.  3.15 a, ω values are shown for Cu-OFP at the transition from the work hardening to the creep range. There is temperature dependence but only a weak influence of the strain rate.

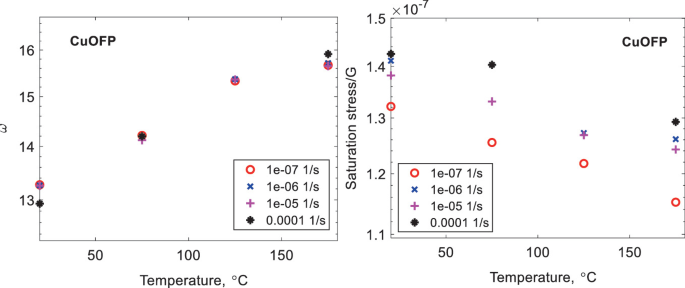
Voce parameters for tensile stress strain curves of Cu-OFP as a function of temperatures for four strain rates; a dynamic recovery parameter ω; b saturation stress σ sat divided by the shear modulus G . Data from [ 3 ]

According to Eq. ( 3.13 ), there is a close relation between ω and the saturation stress σ sat .

Many times the yield strength σ y is only a small fraction of σ sat and then ω is roughly inversely proportional to σ sat . As can be seen for Cu-OFP in Fig.  3.15 b the temperature dependence of σ sat is inverted in comparison to that of ω. However, the strain rate effect is much larger for σ sat in comparison to that of ω. The reason is that the yield strength depends on the strain rate and that has a direct influence on σ sat .

In the literature σ sat has been studied more frequently than ω. In principle, this should not make much difference since σ sat and ω are related according to Eq. ( 3.28 ). However, in a number of cases it has not been checked whether the data points are in the work hardening or in the creep range. If attempts to determine σ sat are made in the creep range, it is in fact σ creep that has been measured, the value of σ sat would be strongly underestimated and the role of dynamic recovery cannot be assessed. Data from [ 13 ] have been used in several influential papers to discuss the temperature dependence of dynamic recovery [ 10 , 14 ]. However, most of the data in [ 13 ] are in the creep range and a significant fraction even in the high temperature range. This has probably contributed to the misconception that dynamic recovery is strongly temperature and strain rate dependent.

Even with the modest temperature dependence of ω that has been observed, Figs.  3.13 and 3.14 , it is natural to assume that dynamic recovery is a thermally activated process, and an Arrhenius expression is formulated

ω RT is the ω value at room temperature that is determined with the help of Eq. ( 3.28 ). Q ω is an activation energy and T RT room temperature. Expression ( 3.29 ) is compared to the available experiments for fcc alloys for ω in Fig.  3.16 .

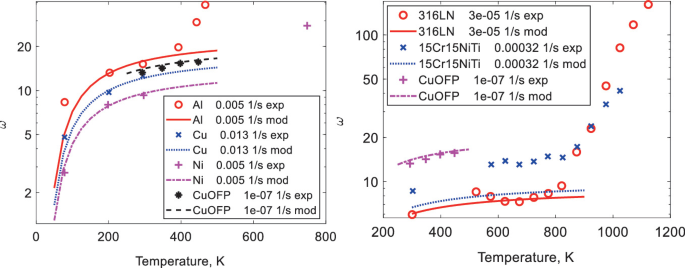
The dynamic recovery parameter ω as a function of temperature for fcc materials. Data from [ 1 , 11 , 15 ]

By fitting Eq. ( 3.29 ) to the experimental data, a value of Q ω  = 1 kJ/mol was found that is two orders of magnitude smaller than typical activation energies for self-diffusion. Values for the pure elements Al, Cu and Ni are included in Fig.  3.16 a from [ 15 ]. The values from [ 15 ] had to be corrected due to numerical errors in the paper, which makes the values uncertain. The same value of Q ω could be used for the six materials in Fig.  3.16 . The expression in Eq. ( 3.29 ) is of course only valid below the creep range.

Since the 1950ties there has been an almost unanimous opinion in the literature that cross-slip is the controlling mechanism for dynamic recovery, see for example [ 10 , 16 , 17 ]. Notable exceptions are [ 18 , 19 ]. Cross-slip has been observed many times during work hardening. However, cross-slip is associated with high activation energies. Modern ab initio calculations give values from 50 to 300 kJ/mol, see Sect.  2.6.3 . High energies are required to form the constrictions that are necessary to make the extended screw dislocations in fcc alloys cross-slip. Such high activation energies are clearly at variance with the low value of Q ω found above.

Attempts to quantitatively model cross-slip are rare and apparently not very successful [ 18 ]. The role of cross-slip for dynamic recovery should not be completely ruled out. It is well-known that cross-slip takes place in deformation stage I in fcc single crystals. In this stage the stresses are very low and if high activation energies would be required, cross-slip would simply not take place [ 18 , 19 ]. Another possible mechanism for dynamic recovery is the formation of dislocation tangles on secondary glide planes which is known to take place in early stages of work hardening [ 10 ]. Argon has suggested an entirely different mechanism for dynamic recovery [ 19 ]. As discussed in Sect.  2.4 , gliding dislocations are expected to pass through about two cell boundaries when they are released. When moving through cell boundaries, they would remove dislocation locks and other dislocations configurations from the cell boundaries thus reducing the dislocation content.

If cross-slip of extended dislocations is active it is natural that the stacking fault energy plays a major role. Argon and Moffatt have derived an expression for how much the climb rate for extended dislocations is slowed down in comparison to perfect dislocations [ 20 ]

γ SFE is the stacking fault energy. The main factor in this expression is the dimensionless quantity \(\left( {\frac{{\upgamma _{{{\text{SFE}}}} }}{Gb}} \right)^{2}\) . The factor 8 was originally 24, but has been changed due to an error in [ 20 ]. The influence on gliding dislocations is the same as for climbing ones since it is the jogs on the dislocations that control their speed, see Sect.  2.6.2 . The three elements in Fig.  3.16 have quite different values of γ SFE . No effect of γ SFE is thus observed in the Figure. However, the shear modulus seems to have an impact. In Fig.  3.14 , the predicted ω value is also divided by the shear modulus squared following Eq. ( 3.30 ). As can be seen ω is approximately proportional to one over the shear modulus squared at least below the creep range. This means that the temperature dependence of ω is of the same type as that of G . The influence of temperature on the shear modulus can be modeled with ab initio methods taking the role of phonons into account. The value of ω during cyclic loading is discussed in Chap. 11 .

3.5 Summary

Stress strain curves are mostly described by well-known empirical models such as Hollomon, Ludwik, Voce and Swift. With the available parameters in these models, there is in general little problem to obtain a good fit to the data. As with most empirical models, it is difficult or impossible to generalize the results. Only the Voce model has been possible to derive from basic principles.

With the basic models for dislocation creep, accurate descriptions of the stress strain curves can be obtained. This is natural since the basic models should be applicable whether the deformation is load, stress or strain rate controlled.

The dynamic recovery parameter ω plays a special role because it describes how the increase in the dislocation stress deviates from a linear behavior and tends to a saturation value. The temperature dependence of ω has been analysed. Although the amount of data is limited, it suggests that ω has the same temperature dependence as the inverse of the shear modulus squared.

It has been proposed in many places in the literature that dynamic recovery is controlled by cross-slip. However, this requires that partial dislocations can be brought together and form a constriction. This would require an activation energy of about 50 kJ/mol or more that would give the dynamic recovery parameter a strong temperature dependence. However, this is in direct conflict with observations, see previous bullet. The role of cross-slip remains unexplained.

B.K. Choudhary, E.I. Samuel, K. Bhanu Sankara Rao, S.L. Mannan, Tensile stress–strain and work hardening behaviour of 316LN austenitic stainless steel. Mater. Sci. Technol. 17 , 223–231 (2001)

Google Scholar  

D.C. Ludwigson, Modified stress-strain relation for FCC metals and alloys. Metall. Trans. 2 , 2825–2828 (1971)

Article   CAS   Google Scholar  

R. Sandström, J. Hallgren, The role of creep in stress strain curves for copper. J. Nucl. Mater. 422 , 51–57 (2012)

Article   Google Scholar  

U.F. Kocks, Laws for work-hardening and low-temperature creep. J. Eng. Mater. Technol. Trans. ASME, 98 Ser H, 76–85 (1976)

Y. Bergström, A dislocation model for the stress-strain behaviour of polycrystalline α-Fe with special emphasis on the variation of the densities of mobile and immobile dislocations. Mater. Sci. Eng. 5 , 193–200 (1970)

Y. Bergström, W. Roberts, A dislocation model for dynamical strain ageing of α-iron in the jerky-flow region. Acta Metall. 19 , 1243–1251 (1971)

F.F. Lavrentev, The type of dislocation interaction as the factor determining work hardening. Mater. Sci. Eng. 46 , 191–208 (1980)

A. Orlová, On the relation between dislocation structure and internal stress measured in pure metals and single phase alloys in high temperature creep. Acta Metall. Mater. 39 , 2805–2813 (1991)

R. Sandstrom, S. Waqas Ahmad, K.T. Pasupuleti, M. Mahdavi Shahri, Slow strain rate tensile testing of friction stir welded Cu-OFP. Constitutive equations for creep, Swedish Nuclear Waste Management Company Report R-13-33 (2017)

U.F. Kocks, H. Mecking, Physics and phenomenology of strain hardening: the FCC case. Prog. Mater Sci. 48 , 171–273 (2003)

P.V. Sivaprasad, S. Venugopal, S. Venkadesan, Tensile flow and work-hardening behavior of a Ti-modified austenitic stainless steel. Metall. Mater. Trans. A 28 , 171–178 (1997)

W.J. Poole, J.D. Embury, D.J. Lloyd, Work hardening in aluminium alloys, in Fundamentals of Aluminium Metallurgy: production, Processing and Applications (2010), pp. 307–344.

H. Mecking, B. Nicklas, N. Zarubova, U.F. Kocks, A “universal” temperature scale for plastic flow. Acta Metall. 34 , 527–535 (1986)

E. Nes, K. Marthinsen, Modeling the evolution in microstructure and properties during plastic deformation of f.c.c.-metals and alloys—An approach towards a unified model. Mater. Sci. Eng.: A 322 , 176–193 (2002)

H. Hallén, A theory of dynamic recovery in F.C.C. metals. Mater. Sci. Eng. 72 , 119–123 (1985)

P.J. Jackson, Dislocation modelling of shear in f.c.c. crystals, Progress in Mater. Sci. 29 , 139–175 (1985)

E. Nes, Modelling of work hardening and stress saturation in FCC metals. Prog. Mater. Sci. 41 , 129–193 (1997)

M. Zaiser, P. Hähner, Stage-III hardening revisited, philosophical magazine a: physics of condensed matter. Struct. Defects Mech. Prop. 77 , 1515–1529 (1998)

A. Argon, Strengthening Mechanisms in Crystal Plasticity (Oxford University Press, Oxford, 2008)

A.S. Argon, W.C. Moffatt, Climb of extended edge dislocations. Acta Metall. 29 , 293–299 (1981)

Download references

Author information

Authors and affiliations.

Material Science and Engineering, Royal Institute of Technology, Stockholm, Sweden

Rolf Sandström

You can also search for this author in PubMed   Google Scholar

Corresponding author

Correspondence to Rolf Sandström .

Rights and permissions

Open Access This chapter is licensed under the terms of the Creative Commons Attribution 4.0 International License ( http://creativecommons.org/licenses/by/4.0/ ), which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license and indicate if changes were made.

The images or other third party material in this chapter are included in the chapter's Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the chapter's Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder.

Reprints and permissions

Copyright information

© 2024 The Author(s)

About this chapter

Sandström, R. (2024). Stress Strain Curves. In: Basic Modeling and Theory of Creep of Metallic Materials. Springer Series in Materials Science, vol 339. Springer, Cham. https://doi.org/10.1007/978-3-031-49507-6_3

Download citation

DOI : https://doi.org/10.1007/978-3-031-49507-6_3

Published : 10 January 2024

Publisher Name : Springer, Cham

Print ISBN : 978-3-031-49506-9

Online ISBN : 978-3-031-49507-6

eBook Packages : Chemistry and Materials Science Chemistry and Material Science (R0)

Share this chapter

Anyone you share the following link with will be able to read this content:

Sorry, a shareable link is not currently available for this article.

Provided by the Springer Nature SharedIt content-sharing initiative

• Publish with us

Policies and ethics

• Find a journal
• Track your research

Academia.edu no longer supports Internet Explorer.

To browse Academia.edu and the wider internet faster and more securely, please take a few seconds to  upgrade your browser .

Enter the email address you signed up with and we'll email you a reset link.

• We're Hiring!
• Help Center

Download Free PDF

Stress-strain curve of paper revisited

2012, Nordic Pulp and Paper Research Journal

We have investigated a relation between micromechanical processes and the stress-strain curve of a dry fiber network during tensile loading. By using a detailed particle-level simulation tool we investigate, among other things, the impact of “non-traditional” bonding parameters, such as compliance of bonding regions, work of separation and the actual number of effective bonds. This is probably the first three-dimensional model which is capable of simulating the fracture process of paper accounting for nonlinearities at the fiber level and bond failures. The failure behavior of the network considered in the study could be changed significantly by relatively small changes in bond strength, as compared to the scatter in bonding data found in the literature. We have identified that compliance of the bonding regions has a significant impact on network strength. By comparing networks with weak and strong bonds, we concluded that large local strains are the precursors of bond failures and ...

Related papers

Despite their obvious importance for theproperties of paper, relatively little is known on thedeformation and damage mechanisms of inter-fibrebonds, compared to the fibres. In order to tailor theproperties of fibre network materials, bybiochemical and/or mechanical modifications of thefibre wall and fibre surfaces, better understandingon how to evaluate the properties of the bonds atthe microscopic level is desirable. This paperreports on the development of a method includingmanufacturing of fibre-fibre cross test pieces, setupand analysis of experimental results. Evaluationof the strength of modified and non-modified kraftpulp fibres has been investigated. The measuredload-displacement responses have been analyzednumerically, taking into account the initial curvedshape of the crossing fibres.Of particular interest has been to estimate the stateof loading in terms of normal and shear componentsin the bond region at failure. Different modes ofbond failure have been observed in the ex...

Nordic Pulp &amp; Paper Research Journal, 2016

Scientific Reports, 2013

Mechanics of Materials, 2012

International Journal of Solids and Structures

Nordic Pulp and Paper Research Journal, 2012

The structure of paper influences its properties and simulations of it are necessary to understand the impact of fiber and papermaking conditions on the sheet properties. We show a method to develop a representative structure of paper by merging different simulation techniques for the forming section and the pressing operation. The simulation follows the bending and drape of fibers over one another in the final structure and allows estimation of sheet properties without recourse to arbitrary bending rules or experimental measurements of density and/or RBA. Fibers are first modeled as jointed beams following the fluid mechanics in the forming section. The sheet structure obtained from this is representative of the wet sheet from the couch. The pressing simulation discretizes fibers into a number of solid elements around the lumen. Bonding between fibers is simulated using spring elements. The resulting fiber network was analyzed to determine its elastic modulus and deformation under ...

Engineering Fracture Mechanics, 2014

Composites Science and Technology, 2005

El Mundo (16 Agosto), 2024

Kelten. Jaarboek Stichting A. G. van Hamel voor Keltische Studies., 2022

Mediaevalia, textos e estudos, 2019

Bezpieczne czy zniewolone? Szkice o zwierzętach, red. H. Mamzer, A. Żok, Oficyna Wydawnicza Epigram, Bydgoszcz 2019, s. 249-277., 2019

Cognitive Linguistics and Second Language Acquisition of Chinese, 2024

Genomics, 1991

PloS one, 2014

Siti Masrurotul Hasanah , 2024

Agriculture and Human Values, 2002

Interface - Comunicação, Saúde, Educação, 2021

European Scientific Journal, ESJ, 2018

International Journal for Research in Applied Science and Engineering Technology, 2022

Epígrafe, 2021

Related topics

•   We're Hiring!
•   Help Center
• Find new research papers in:
• Health Sciences
• Earth Sciences
• Cognitive Science
• Mathematics
• Computer Science
• Academia ©2024

• Architecture and Design
• Asian and Pacific Studies
• Business and Economics
• Classical and Ancient Near Eastern Studies
• Computer Sciences
• Cultural Studies
• Engineering
• General Interest
• Geosciences
• Industrial Chemistry
• Islamic and Middle Eastern Studies
• Jewish Studies
• Library and Information Science, Book Studies
• Life Sciences
• Linguistics and Semiotics
• Literary Studies
• Materials Sciences
• Mathematics
• Social Sciences
• Sports and Recreation
• Theology and Religion
• Publish your article
• The role of authors
• Promoting your article
• Abstracting & indexing
• Publishing Ethics
• Why publish with De Gruyter
• How to publish with De Gruyter
• Our book series
• Our subject areas
• Your digital product at De Gruyter
• Contribute to our reference works
• Product information
• Tools & resources
• Product Information
• Promotional Materials
• Orders and Inquiries
• FAQ for Library Suppliers and Book Sellers
• Repository Policy
• Free access policy
• Open Access agreements
• Database portals
• For Authors
• Customer service
• People + Culture
• Journal Management
• How to join us
• Working at De Gruyter
• Mission & Vision
• De Gruyter Foundation
• De Gruyter Ebound
• Our Responsibility
• Partner publishers

Your purchase has been completed. Your documents are now available to view.

Stress-strain curve of paper revisited

We have investigated a relation between micromechanical processes and the stress-strain curve of a dry fiber network during tensile loading. By using a detailed particle-level simulation tool we investigate, among other things, the impact of “non-traditional” bonding parameters, such as compliance of bonding regions, work of separation and the actual number of effective bonds. This is probably the first three-dimensional model which is capable of simulating the fracture process of paper accounting for nonlinearities at the fiber level and bond failures. The failure behavior of the network considered in the study could be changed significantly by relatively small changes in bond strength, as compared to the scatter in bonding data found in the literature. We have identified that compliance of the bonding regions has a significant impact on network strength. By comparing networks with weak and strong bonds, we concluded that large local strains are the precursors of bond failures and not the other way around.

© 2018 by Walter de Gruyter Berlin/Boston

• X / Twitter

Supplementary Materials

Please login or register with De Gruyter to order this product.

Journal and Issue

Articles in the same issue.