example of problem solving in geometric sequence

• The Basic math blog
• Member Login
• Pre-Algebra Lessons
• Pre-Algebra Word Problems
• Pre-Algebra Calculators
• Algebra Lessons
• Algebra Word Problems
• Algebra Proofs
• Advanced Algebra
• Algebra Calculators
• Geometry Lessons
• Geometry Word Problems
• Geometry Proofs
• Geometry Calculators
• Trigonometry Lessons
• Numeration System
• Basic Concepts of Set Theory
• Consumer Math
• Baseball Math
• Math for Nurses
• Statistics Made Easy
• High School Physics
• Basic Mathematics Store
• Math Vocabulary Quizzes
• SAT Math Prep
• Math Skills by Grade Level
• Ask an Expert
• Other Websites
• K-12 Worksheets
• Worksheets Generator
• Algebra Worksheets
• Geometry Worksheets
• Fun Online Math Games
• Pre-Algebra Games
• Math Puzzles
• Math Tricks

Geometric sequence word problems

This lesson will show you how to solve a variety of geometric sequence word problems.

Example #1:

The stock's price of a company is not doing well lately. Suppose the stock's price is 92% of its previous price each day. What is the stock's price after 10 days if the stock was worth $2500 right before it started to go down?  

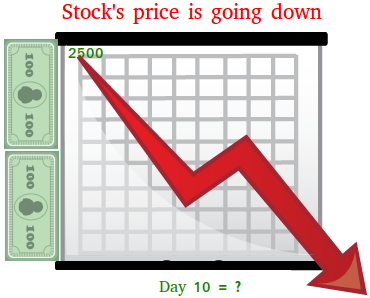
To solve this problem, we need the geometric sequence formula shown below.

a n  = a 1  × r (n - 1)

a 1  = original value of the stock  = 2500

a 2  = value of the stock after 1 day

a 11  = value of the stock after 10 days

a 11 = 2500 × (0.92) (11 - 1)

a 11  = 2500 × (0.92) 10

a 11  = 2500 × 0.434

a 11  = $1085

The stock's price is about 1085 dollars.

Example #2:

The third term of a geometric sequence is 45 and the fifth term of the geometric sequence is 405. If all the terms of the sequence are positive numbers, find the 15th term of the geometric sequence.

Solution To solve this problem, we need the geometric sequence formula shown below.

a n  = a 1  × r (n - 1)

Find the third term

a 3  = a 1  × r (3 - 1)

a 3  = a 1  × r 2

Since the third term is 45,  45 = a 1  × r 2 ( equation 1 )

Find the fifth term

a 5 = a 1  × r (5 - 1)

a 5  = a 1  × r 4

Since the fifth term is 405,  405 = a 1  × r 4 ( equation 2 )

Divide equation 2 by equation 1 .

(a 1  × r 4 ) / (a 1  × r 2 ) = 405 / 45

Cancel a 1 since it is both on top and at the bottom of the fraction.

r 4 / r 2 = 9

r = ±√9

r = ±3

Use r  = 3, and equation 1 to find a 1

45 = a 1  × (3) 2

45 = a 1  × 9

a 1 = 45 / 9 = 5

Since all the terms of the sequence are positive numbers, we must use r = 3 if we want all the terms to be positive numbers.

Let us now find a 15

a 15 = 5 × (3) (15 - 1)

a 15 = 5 × (3) 14  

a 15  = 5 × 4782969 

a 15  =  23914845

Challenging geometric sequence word problems

Example #3:

Suppose that the magnification of a PDF file on a desktop computer is increased by 15% for each level of zoom. Suppose also that the original length of the word " January " is 1.2 cm. Find the length of the word " January " after 6 magnifications.

a 1  = original length of the word  = 1.2 cm

a 2 = length of the word after 1 magnification

a 7 = length of the word after 6 magnifications

r = 1 + 0.15 = 1.15

a 7  = 1.2 × (1.15) (7 - 1)

a 7 = 1.2 × (1.15) 6

a 7 = 1.2 × 2.313

a 7  = 2.7756

After 6 magnifications, the length of the word "January" is 2.7756 cm.

Notice that we added 1 to 0.15. Why did we do that? Let us not use the formula directly so you can see the reason behind it. Study the following carefully !

Day 1 : a 1 = 1.2

Day 2 : a 2 = 1.2 + 1.2 (0.15) = 1.2 (1 + 0.15)

Day 3 : a 3 =  1.2(1 + 0.15) + [ 1.2(1 + 0.15) ]0.15 =  1.2(1 + 0.15) (1 + 0.15) = 1.2(1 + 0.15) 2

Day 7 : a 7 = 1.2(1 + 0.15) 6

Suppose that you want a reduced copy of a photograph. The actual length of the photograph is 10 inches. If each reduction is 64% of the original, how many reductions, will shrink the photograph to 1.07 inches.

a 1  = original length of the photograph  = 10 inches

a 2  = length of the photograph after 1 reduction

n = number of reductions = ?

1.07 = 10 × (0.64) (n - 1)

Divide both sides by 10

1.07 / 10 = [10 × (0.64) (n - 1) ] / 10

0.107 = (0.64) (n - 1)

Notice that you have an exponential equation to solve. The biggest challenge then is knowing how to solve exponential equations !

Take the natural log of both sides of the equation.

ln(0.107) = ln[(0.64) (n - 1) ]

Use the power property of logarithms .

ln(0.107) = (n - 1)ln(0.64)

Divide both sides of the equation by ln(0.64)

ln(0.107) / ln(0.64) = (n - 1)ln(0.64) / ln(0.64)

n - 1 = ln(0.107) / ln(0.64)

Use a calculator to find ln(0.107) and ln(0.64)

n - 1 = -2.23492644452 \ -0.44628710262

n - 1 = 5.0078

n = 1 + 5.0078

Therefore, you will need 6 reductions.

Geometric sequence

Arithmetic sequence word problems

Special Math Topics

Applied math.

About me :: Privacy policy :: Disclaimer :: Donate   Careers in mathematics  

Copyright © 2008-2021. Basic-mathematics.com. All right reserved

• Mathematics
• Number System and Arithmetic
• Trigonometry
• Probability
• Mensuration
• Maths Formulas

Practice Problems on Geometric Series

A  geometric series  is a type of infinite series formed by summing the terms of a geometric sequence. A geometric sequence is a sequence of numbers where each term after the first is found by multiplying the previous term by a fixed, non-zero number called the  common ratio .

The general form of a geometric series can be expressed as:

S = a + ar + ar^2 + ar^3 + ar^4 + \ldots

• S is the sum of the series.
• a is the first term.
• r is the common ratio.

Sample Questions of Geometric Series

Question 1: What is the Geometric mean 2, 4, 8?

Solution:  

According to the formula,  =\sqrt [3]{(2)(4)(8)}\\=4

Question 2: Find the first term and common factor in the following Geometric Progression:

4, 8, 16, 32, 64, . . .

Here, It is clear that the first term is 4, a=4 We obtain common Ratio by dividing 1st term from 2nd: r = 8/4 = 2

Question 3: Find the 8 th and the n th term for the G.P: 3, 9, 27, 81, . . .

Solution: 

Put n=8 for 8 th term in the formula: ar n-1 For the G.P : 3, 9, 27, 81 . . . First term (a) = 3 Common Ratio (r) = 9/3 = 3 8 th term = 3(3) 8-1 = 3(3) 7 = 6561 N th = 3(3) n-1 = 3(3) n (3) -1 = 3 n

Question 4: For the G.P. : 2, 8, 32, . . . which term will give the value 131073?

Assume that the value 131073 is the N th term, a = 2, r = 8/2 = 4 N th term (a n ) = 2(4) n-1 = 131073 4 n-1 = 131073/2 = 65536 4 n-1 = 65536 = 4 8 Equating the Powers since the base is same: n-1 = 8 n = 9

Question 5: Find the sum up to 5 th and N th term of the series:  1, \frac{1}{2},\frac{1}{4},\frac{1}{8}...

a= 1, r = 1/2 Sum of N terms for the G.P,  {S_n =\frac{a(1-r^n)}{1-r}}                                                  = \frac{1(1-(\frac{1}{2})^n)}{1-\frac{1}{2}}   = 2 (1-(\frac{1}{2})^n) Sum of first 5 terms ⇒ a 5 =  2 ( 1-(\frac{1}{2})^5) =  2 ( 1-(\frac{1}{32})) =  (\frac{31}{16})

Question 6: Find the Sum of the Infinite G.P: 0.5, 1, 2, 4, 8, ...

Formula for the Sum of Infinite G.P:  \frac{a}{1-r}  ; r≠0 a = 0.5, r = 2 S ∞ = (0.5)/(1-2) = 0.5/(-1)= -0.5

Question 7: Find the sum of the Series: 5, 55, 555, 5555,... n terms

Solution : 

The given Series is not in G.P but it can easily be converted into a G.P with some simple modifications. Taking 5 common from the series: 5 (1, 11, 111, 1111,... n terms) Dividing and Multiplying with 9:  \frac{5}{9}(9+ 99+ 999+...n terms) ⇒  \frac{5}{9}[((10+(10)^2+(10)^3+...n terms)-(1+1+1+...n terms)] ⇒ \frac{5}{9}[(\frac{10((10)^n-1)}{10-1})-(n)] ⇒  \frac{5}{9}[(\frac{10((10)^n-1)}{9})-(n)]

Worksheet: Geometric Series

You can download this free worksheet with answer key from follows:

• Sequence and Series
• Geometric Series
• Sum of Infinite Geometric Series
• Arithmetic Series

Similar Reads

• School Learning
• Practice Questions

Improve your Coding Skills with Practice

What kind of Experience do you want to share?

GCSE Tutoring Programme

Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring.

In order to access this I need to be confident with:

This topic is relevant for:

Geometric Sequences

Here we will learn what a geometric sequence is, how to continue a geometric sequence, how to find missing terms in a geometric sequence, and how to generate a geometric sequence.

At the end, you’ll find geometric sequence worksheets based on Edexcel, AQA, and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is a geometric sequence?

A geometric sequence (geometric progression) is an ordered set of numbers that progresses by multiplying or dividing each term by a common ratio.

If we multiply or divide by the same number each time to make the sequence, it is a geometric sequence .

The common ratio is the same for any two consecutive terms in the same sequence.

Here are a few examples,

What are geometric sequences?

Geometric sequence formula

The geometric sequence formula is,

Where, 

\pmb{ a_{n} } is the n^{th} term (general term),

\pmb{ a_{1} } is the first term,

\pmb{ n } is the term position,

and \pmb{ r } is the common ratio.

We get the geometric sequence formula by looking at the following example,

We can see the common ratio (r) is 2 , so r = 2 .

a_{1} is the first term which is 5 ,

a_{2} is the second term which is 10 ,

and a_{3} is the third term which is 20 etc.

However we can write this using the common difference of 2 ,

Related lessons on sequences

Geometric sequences  is part of our series of lessons to support revision on  sequences . You may find it helpful to start with the main sequences lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

• Quadratic sequences
• Arithmetic sequence
• Nth term of a sequence
• Recurrence relation
• Quadratic nth term

How to continue a geometric sequence

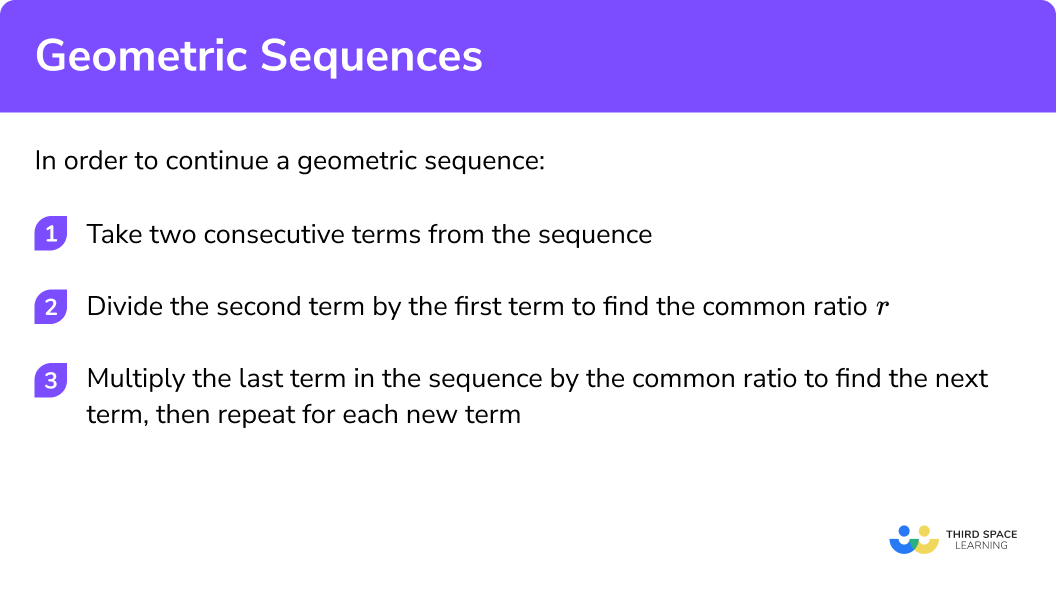
To continue a geometric sequence, you need to calculate the common ratio. This is the factor that is used to multiply one term to get the next term. To calculate the common ratio and continue a geometric sequence you need to:

Take two consecutive terms from the sequence.

• Divide the second term by the first term to find the common ratio r .

Multiply the last term in the sequence by the common ratio to find the next term. Repeat for each new term.

Explain how to continue a geometric sequence

Geometric sequences worksheet

Get your free geometric sequences worksheet of 20+ questions and answers. Includes reasoning and applied questions.

Continuing a geometric sequence examples

Example 1: continuing a geometric sequence.

Calculate the next three terms for the geometric progression 1, 2, 4, 8, 16, …

Here we will take the numbers 4 and 8 .

2 Divide the second term by the first term to find the value of the common ratio, r .

3 Multiply the last term in the sequence by the common ratio to find the next term. Repeat for each new term.

The next three terms in the sequence are 32, 64, and 128 .

Example 2: continuing a geometric sequence with negative numbers

Calculate the next three terms for the sequence -2, -10, -50, -250, -1250, …

Here we will take the numbers -10 and -50 .

Divide the second term by the first term to find the common ratio, r.

The next three terms are -6250, -31250, and -156250.

Example 3: continuing a geometric sequence with decimals

Calculate the next three terms for the sequence 100, 10, 1, 0.1, 0.01, …

Here we will take the numbers 0.1 and 0.01 .

Divide the second term by the first term to find the common ratio, r .

The next three terms in the sequence are 0.001, 0.0001, and 0.00001.

Example 4: continuing a geometric sequence involving fractions

Calculate the next three terms for the sequence

Here we will take the numbers 5 and 2\frac{1}{2} .

The next three terms are

\frac{5}{16}, \frac{5}{32}, and \frac{5}{64} .

 Geometric sequence practice questions – continue the sequence

1. Write the next three terms of the sequence 0.5, 5, 50, 500, …

Choose two consecutive terms. For example, 5 and 50 .

Common ratio,

2. Write the next three terms of the sequence 0.04, 0.2, 1, 5, 25, …

Choose two consecutive terms. For example, 5 and 25 .

3. Calculate the next 3 terms of the sequence -1, -3, -9, -27, -81, …

Choose two consecutive terms. For example, -27 and -9 .

4. By finding the common ratio, state the next 3 terms of the sequence 640, 160, 40, 10, 2.5 .

Choose two consecutive terms. For example, 40 and 10 .

5. Work out the common ratio and therefore the next three terms in the sequence 36, 12, 4, \frac{4}{3}, \frac{4}{9}, …

Choose two consecutive terms. For example, 12 and 4 .

6. Find the common ratio and hence calculate the next three terms of the sequence 1, -1, 1, -1, 1, …

Choose two consecutive terms. For example, -1 and 1 .

How to find missing numbers in a geometric sequence

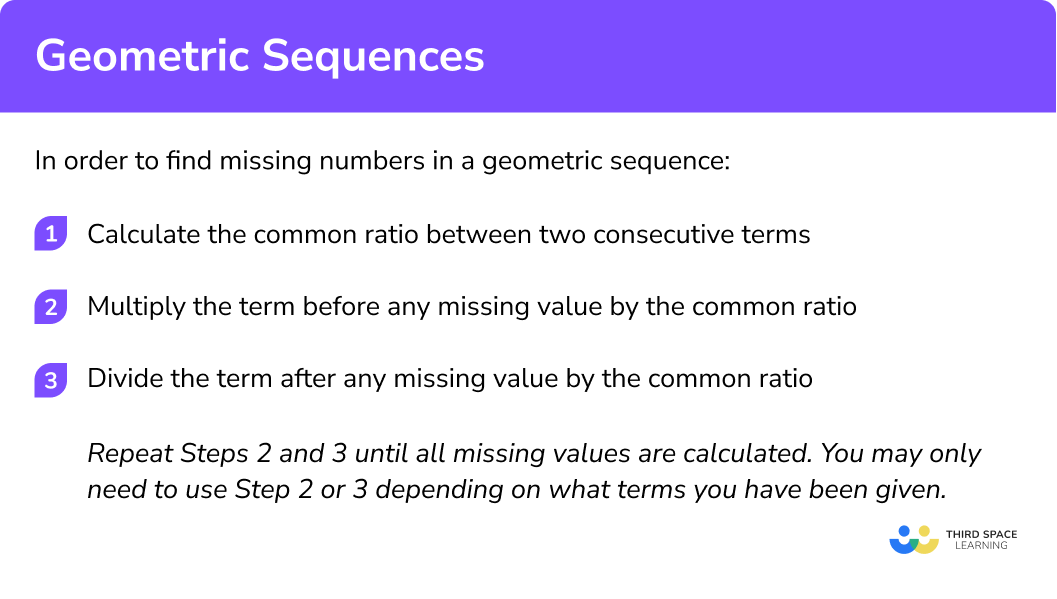
The common ratio can be used to find missing numbers in a geometric sequence. To find missing numbers in a geometric sequence you need to:

Calculate the common ratio between two consecutive terms.

• Multiply the term before any missing value by the common ratio.

Divide the term after any missing value by the common ratio.

Repeat Steps 2 and 3 until all missing values are calculated. You may only need to use Step 2 or 3 depending on what terms you have been given.

Explain how to find missing numbers in a geometric sequence

Finding missing numbers in a geometric sequence examples

Example 5: find the missing numbers in the geometric sequence.

Fill in the missing terms in the sequence 7, 14, …, …,112 .

  Multiply the term before any missing value by the common ratio.

The missing terms are 28 and 56 .

Note: Here, you could repeat Step 2 by using 28 \times 2 = 56.

Example 6: find the missing numbers in a geometric sequence including decimals

Find the missing values in the sequence 0.4, …, ..., 137.2, 960.4.

  Divide the term after any missing value by the common ratio.

The missing terms are 2.8 and 19.6 .

Example 7: find the missing numbers in a geometric sequence when there are multiple consecutive terms missing

Find the missing values in the sequence, -4, ..., …, -108,...

First, we need to find the factor between the two terms, -108 \div -4 = 27 .

To get from -108 to -4 , we jump 3 terms.

This means that -4 has been multiplied by the common ratio three times or -4 \times r \times r \times r = -4r^3 .

\begin{aligned} r^{3}&=27\\\\ r&=3 \end{aligned}

Note: Term -108 is already given.

The missing terms are -12, -36, and -324.

We don’t need to complete this step.

Example 8: find the missing numbers in a geometric sequence including mixed numbers

Find the missing values in the sequence

  Calculate the common ratio between two consecutive terms.

Repeat this step to find the next term.

40 \frac{1}{2} \times 3=121 \frac{1}{2}

The missing terms in the sequence are

1 \frac{1}{2}, 40 \frac{1}{2}, and 121 \frac{1}{2} .

 Geometric sequence practice questions – find missing numbers

1. Find the missing numbers in the geometric sequence 4, 2, …, 0.5, …

Choose two consecutive terms. For example, 4 and 2 .

2. Find the missing numbers in the sequence -7, -35, …, …, -4375

Choose two consecutive terms. For example, -7 and -35 .

3. Find the missing terms in the sequence 0.6, …, …, 0.075, 0.0375

Choose two consecutive terms. For example, 0.075 and 0.0375 .

4. Calculate the missing terms in the arithmetic sequence 2 \frac{1}{5}, \frac{11}{20}, \frac{11}{80}, \ldots, \ldots

Choose two consecutive terms. For example, \frac{11}{20} and \frac{11}{80} .

5. Work out the missing terms in the sequence 3, …, …, 24 .

3 has been multiplied by the common ratio, r, three times to get 24.

3 \times r \times r \times r=24 \text{ or } 3r^{3}=24 .

Solving the equation,

6. Work out the missing terms in the sequence 90, …, …, \frac{10}{3} .

90 has been multiplied by the common ratio, r, three times to get \frac{10}{3}.

90 \times r \times r \times r=\frac{10}{3} \text{ or } 90r^{3}=\frac{10}{3} .

How to generate a geometric sequence

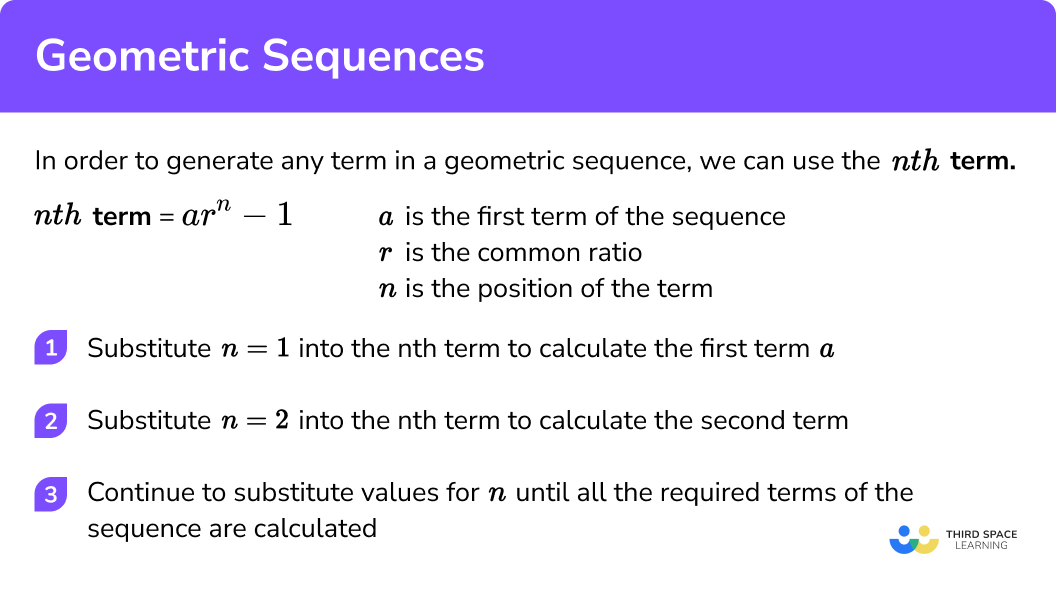
In order to generate a geometric sequence, we need to know the n^{th} term. Using a as the first term of the sequence, r as the common ratio and n to represent the position of the term, the n^{th} term of a geometric sequence is written as ar^{n-1}.

Once we know the first term and the common ratio, we can work out any number of terms in the sequence.

The first term is found when n=1 , the second term when n=2 , the third term when n=3 and so on.

To generate a geometric sequence you need to:

• Substitute n=1 into the n^{th} term to calculate the first term.
• Substitute n=2 into the n^{th} term to calculate the second term.

Continue to substitute values for n until all the required terms of the sequence are calculated.

Explain how to generate a geometric sequence

Generating a geometric sequence examples

Example 9: generate a geometric sequence using the n th term.

Generate the first 5 terms of the sequence 4^{n-1} .

Substitute n = 1 into the n^{th} term to calculate the first term.

When n = 1,\quad 4^{1-1} = 4^{0} = 1 .

  Substitute n = 2 into the n^{th} term to calculate the second term.

When n = 2,\quad 4^{2-1 }= 4^{1} = 4 .

When n=3, \quad 4^{3-1}=4^{2}=16 .

When n=4, \quad 4^{4-1}=4^{3}=64 .

When n=5, \quad 4^{5-1}=4^{4}=256 .

The first 5 terms of the sequence are 1, 4, 16, 64, 256.

Example 10: generate a geometric sequence using a table

Complete the table for the first 5 terms of the arithmetic sequence 6 \times 2^{n-1}.

Example 11: generate larger terms in a geometric sequence

A geometric sequence has the n^{th} term \left(\frac{1}{2}\right)^{n} .

Calculate the 1^{st}, 2^{nd}, 10^{th} and 12^{th} terms in the sequence. Express your answers as fractions. 

When n=1,\quad \left(\frac{1}{2}\right)^{1}=\frac{1}{2} .

Substitute n = 2 into the n^{th} term to calculate the second term.

When n=2, \quad \left(\frac{1}{2}\right)^{2}=\frac{1}{4} .

When n=10,\quad \left(\frac{1}{2}\right)^{10}=\frac{1}{1024} .

When n=12,\quad \left(\frac{1}{2}\right)^{12}=\frac{1}{4096} .

The unknown terms are

1, \frac{1}{4}, \frac{1}{1024}, and \frac{1}{4096} .

Example 12: generate a geometric sequence with a negative common ratio

Generate the first 5 terms of the geometric sequence 2(- 3)^{n-1} .

When n=1, \quad 2(-3)^{n-1}=2(-3)^{1-1}=2(-3)^{0}=2 \times 1=2 .

When n=2,\quad 2(-3)^{n-1}=2(-3)^{2-1}=2(-3)^{1}=2 \times-3=-6 .

When n=3, \quad 2(-3)^{n-1}=2(-3)^{3-1}=2(-3)^{2}=2 \times 9=18 .

When n=4, \quad 2(-3)^{n-1}=2(-3)^{4-1}=2(-3)^{3}=2 \times-27=-54 .

When n=5, \quad 2(-3)^{n-1}=2(-3)^{5-1}=2(-3)^{4}=2 \times 81=162 .

The first 5 terms of the sequence are 2, -6, 18, -54, and 162 .

 Geometric sequence practice questions – generate a sequence

1. Generate the first 5 terms of the sequence 10^{n} .

When  n=1, 10^{1}=10 .

When n=2, 10^{2}=100 .

When n=3, 10^{3}=1000 .

When n=4, 10^{4}=10000 .

When n=5, 10^{5}=100000 .

2. Generate the first 5 terms of the sequence 5^{n-1} .

When n=1, 5^{1-1}=5^{0}=1 .

When n=2, 5^{2-1}=5^{1}=5 .

When n=3, 5^{3-1}=5^{2}=25 .

When n=4, 5^{4-1}=5^{3}=125 .

When n=5, 5^{5-1}=5^{4}=625  .

3. Generate the first 5 terms of the sequence 4 \times 3^{n-1} .

When n=1, 4 \times 3^{1-1}=4 \times 3^{0}=4 .

When n=2, 4 \times 3^{2-1}=4 \times 3^{1}=12 .

When n=3, 4 \times 3^{3-1}=4 \times 3^{2}=36 .

When n=4, 4 \times 3^{4-1}=4 \times 3^{3}=108 .

When n=5, 4 \times 3^{5-1}=4 \times 3^{4}=324  .

4. Generate the first 5 terms of the sequence \frac{3^{n}}{6} .

When n=1, \frac{3^1}{6}= \frac{1}{2} .

When n=2, \frac{3^2}{6}= \frac{9}{6} = 1 \frac{1}{2} .

When n=3, \frac{3^3}{6}= \frac{27}{6} = 4 \frac{1}{2} .

When n=4, \frac{3^4}{6}= \frac{81}{6} = 13 \frac{1}{2} .

When n=5, \frac{3^5}{6}= \frac{243}{6} = 40 \frac{1}{2}  .

5. Calculate the 1st, 3rd, 10th and 15th term of the sequence 2^{n} .

When n=1, 2^{1}= 2 .

When n=3, 2^{3}= 8 .

When n=10, 2^{10}= 1024 .

When n=15, 2^{15}= 32768 .

6. Calculate the first 5 terms of the sequence 3 \times (-5)^{n-1} .

When n=1, 3 \times (-5)^{1-1} = 3 \times (-5)^{0} = 3 .

When n=2, 3 \times (-5)^{2-1} = 3 \times (-5)^{1} = -15 .

When n=3, 3 \times (-5)^{3-1} = 3 \times (-5)^{2} = 75 .

When n=4, 3 \times (-5)^{4-1} = 3 \times (-5)^{3} = -375 .

When n=5, 3 \times (-5)^{5-1} = 3 \times (-5)^{4} = 1875 .

Geometric sequences GCSE exam questions

1. Which sequence is a geometric progression?

1, 3, 5, 7, 9,…. \quad \quad \quad 1, 3, 9, 27, 81, …..

1, 3, 6, 10, 15, …. \quad \quad 1, 0.6, 0.2, -0.2, -0.6,….

1, 3, 9, 27, 81, …..

2.  Here is a geometric progression,

1, -5, 25, …., 625, …

(a) Find the common ratio.

(b) Work out the fourth term of the sequence.

25 \div -5 = – 5

Common ratio = -5

25 \times -5

3.  A scientist is studying a type of bacteria. The number of bacteria over the first four days is shown below.

How many bacteria will there be on day 7?

180 \div 60 = 3

1620 \times 3 \times 3 \times 3

Common misconceptions

• Mixing up the common ratio with the common difference for arithmetic sequences

Although these two phrases are similar, each successive term in a geometric sequence of numbers is calculated by multiplying the previous term by a common ratio and not by adding a common difference.

• A negative value for r means that all terms in the sequence are negative

This is not always the case as when r is raised to an even power, the solution is always positive.

• The first term in a geometric sequence

The first term is a . With ar^{n-1} , the first term would occur when n = 1 and so the power of r would be equal to 0 . Anything to the power of 0 is equal to 1 , leaving a as the first term in the sequence. This is usually mistaken when a = 1 as it is not clearly noted in the question for example, 2^{n-1} is the same as 1 \times 2^{n-1} .

• Incorrect simplifying of the n th term

For example, 6 \times 3^{n-1} is incorrectly simplified to 18^{n-1} as 6 \times 3 = 18 .

• The difference between an arithmetic and a geometric sequence

Arithmetic sequences are formed by adding or subtracting the same number. Geometric sequences are formed by multiplying or dividing the same number.

Learning checklist

You have now learned how to:

• Recognise geometric sequences

The next lessons are

• Inequalities
• Functions in algebra
• Laws of indices

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.

Find out more about our GCSE maths tuition programme.

Privacy Overview

• Unit Conversions
• Sets and Types of Numbers
• Common Multiple and Divisor
• Fractions and Decimals
• Algebraic Expressions and Polynomials
• Equations and Inequalities
• Arithmetic Sequence
• Geometric Sequence
• Applications of Sequences
• Infinite Series and Sums
• Limit of a Sequence
• Combinatorics
• Probability and Statistics
• Stereometry
• Analytical Geometry
• Limits, Derivatives, Integrals
• Analysis of Functions
• Trig table and chart

• Link Partners
• Privacy Policy

Copyright © 2015-2023 math-exercises.com - All rights reserved. Any use of website content without written permission is prohibited.

• + ACCUPLACER Mathematics
• + ACT Mathematics
• + AFOQT Mathematics
• + ALEKS Tests
• + ASVAB Mathematics
• + ATI TEAS Math Tests
• + Common Core Math
• + DAT Math Tests
• + FSA Tests
• + FTCE Math
• + GED Mathematics
• + Georgia Milestones Assessment
• + GRE Quantitative Reasoning
• + HiSET Math Exam
• + HSPT Math
• + ISEE Mathematics
• + PARCC Tests
• + Praxis Math
• + PSAT Math Tests
• + PSSA Tests
• + SAT Math Tests
• + SBAC Tests
• + SIFT Math
• + SSAT Math Tests
• + STAAR Tests
• + TABE Tests
• + TASC Math
• + TSI Mathematics
• + ACT Math Worksheets
• + Accuplacer Math Worksheets
• + AFOQT Math Worksheets
• + ALEKS Math Worksheets
• + ASVAB Math Worksheets
• + ATI TEAS 6 Math Worksheets
• + FTCE General Math Worksheets
• + GED Math Worksheets
• + 3rd Grade Mathematics Worksheets
• + 4th Grade Mathematics Worksheets
• + 5th Grade Mathematics Worksheets
• + 6th Grade Math Worksheets
• + 7th Grade Mathematics Worksheets
• + 8th Grade Mathematics Worksheets
• + 9th Grade Math Worksheets
• + HiSET Math Worksheets
• + HSPT Math Worksheets
• + ISEE Middle-Level Math Worksheets
• + PERT Math Worksheets
• + Praxis Math Worksheets
• + PSAT Math Worksheets
• + SAT Math Worksheets
• + SIFT Math Worksheets
• + SSAT Middle Level Math Worksheets
• + 7th Grade STAAR Math Worksheets
• + 8th Grade STAAR Math Worksheets
• + THEA Math Worksheets
• + TABE Math Worksheets
• + TASC Math Worksheets
• + TSI Math Worksheets
• + AFOQT Math Course
• + ALEKS Math Course
• + ASVAB Math Course
• + ATI TEAS 6 Math Course
• + CHSPE Math Course
• + FTCE General Knowledge Course
• + GED Math Course
• + HiSET Math Course
• + HSPT Math Course
• + ISEE Upper Level Math Course
• + SHSAT Math Course
• + SSAT Upper-Level Math Course
• + PERT Math Course
• + Praxis Core Math Course
• + SIFT Math Course
• + 8th Grade STAAR Math Course
• + TABE Math Course
• + TASC Math Course
• + TSI Math Course
• + Number Properties Puzzles
• + Algebra Puzzles
• + Geometry Puzzles
• + Intelligent Math Puzzles
• + Ratio, Proportion & Percentages Puzzles
• + Other Math Puzzles

How to Solve Geometric Sequences? (+FREE Worksheet!)

Learn how to solve Geometric Sequence problems using the following step-by-step guide with detailed solutions.

Related Topics

• How to Solve Finite Geometric Series
• How to Solve Infinite Geometric Series
• How to Solve Arithmetic Sequences

Step by step guide to solve Geometric Sequence Problems

• It is a sequence of numbers where each term after the first is found by multiplying the previous item by the common ratio, a fixed, non-zero number. For example, the sequence \(2, 4, 8, 16, 32\), … is a geometric sequence with a common ratio of \(2\).
• To find any term in a geometric sequence use this formula: \(\color{blue}{x_{n}=ar^{(n – 1)}}\)
• \(a =\) the first term , \(r =\) the common ratio , \(n =\) number of items

Geometric Sequences – Example 1:

Given the first term and the common ratio of a geometric sequence find the first five terms of the sequence. \(a_1=3,r=-2\)

Use geometric sequence formula: \(\color{blue}{x_{n}=ar^{(n – 1)}}\) \(→x_{n}=0.8 .(-5)^{n-1}\) If \(n=1\) then: \(x_{1}=3 .(-2)^{1-1}=3 (1)=3\), First Five Terms: \(3,-6,12,-24,48\)

The Absolute Best Books to Ace Pre-Algebra to Algebra II

The Ultimate Algebra Bundle From Pre-Algebra to Algebra II

Geometric sequences – example 2:.

Given two terms in a geometric sequence find the 8th term. \(a_{3}=10\) and \(a_{5}=40\)

Use geometric sequence formula: \(\color{blue}{x_{n}=ar^{(n – 1)}}\) \(→a_{3}=ar^{(3 – 1)}=ar^2=10\) \(x_{n}=ar^{(n – 1)}→a_5=ar^{(5 – 1)}=ar^4=40\) Now divide \(a_{5}\) by \(a_{3}\). Then: \(\frac{a_{5}}{a_{3}} =\frac{ar^4}{ar^2 }=\frac{40}{10}\), Now simplify: \(\frac{ar^4}{ar^2 }=\frac{40}{10}→r^2=4→r=2\) We can find a now: \(ar^2=12→a(2^2 )=10→a=2.5\) Use the formula to find the 8th term: \(x_{n}=ar^{(n – 1)}→a_8=(2.5) (2)^8=2.5(256)=640\)

Geometric Sequences – Example 3:

Given the first term and the common ratio of a geometric sequence find the first five terms of the sequence. \(a_{1}=0.8,r=-5\)

Use geometric sequence formula: \(\color{blue}{x_{n}=ar^{(n – 1)}}\) \(→x_{n}=0.8 .(-5)^{n-1}\) If \(n=1\) then: \(x_{1}=0.8 .(-5)^{1-1}=0.8 (1)=0.8\), First Five Terms: \(0.8,-4,20,-100,500\)

The Best Book to Help You Ace Pre-Algebra

Pre-Algebra for Beginners The Ultimate Step by Step Guide to Preparing for the Pre-Algebra Test

Geometric sequences – example 4:.

Given two terms in a geometric sequence find the 8th term. \(a_3=12\) and \(a_5=48\)

Use geometric sequence formula: \(\color{blue}{x_{n}=ar^{(n – 1)}}\) \(→a_3=ar^{(3 – 1)}=ar^2=12\) \(\color{blue}{x_{n}=ar^{(n – 1)}}\) \(→a_5=ar^{(5 – 1)}=ar^4=48\) Now divide \(a_{5}\) by \(a_{3}\). Then: \(\frac{a_{5}}{a_{3} }=\frac{ar^4}{ar^2}=\frac{48}{12}\), Now simplify: \(\frac{ar^4}{ar^2}=\frac{48}{12}→r^2=4→r=2\) We can find a now: \(ar^2=12→a(2^2 )=12→a=3\) Use the formula to find the \(8^{th}\) term: \(\color{blue}{x_{n}=ar^{(n – 1)}}\) \(→a_{8}=(3) (2)^8=3(256)=768\)

Exercises for Solving Geometric Sequences

Determine if the sequence is geometric. if it is, find the common ratio..

• \(\color{blue}{1, – 5, 25, – 125, …}\)
• \(\color{blue}{– 2, – 4, – 8, – 16, …}\)
• \(\color{blue}{4, 16, 36, 64, …}\)
• \(\color{blue}{– 3, – 15, – 75, – 375, …}\)

Download Geometric Sequences Worksheet

• \(\color{blue}{r=-5}\)
• \(\color{blue}{r=2}\)
• \(\color{blue}{not \ geometric}\)
• \(\color{blue}{r=5}\)

The Greatest Books for Students to Ace the Algebra

Pre-Algebra Exercise Book A Comprehensive Workbook + PreAlgebra Practice Tests

Pre-algebra in 10 days the most effective pre-algebra crash course, college algebra practice workbook the most comprehensive review of college algebra, high school algebra i a comprehensive review and step-by-step guide to mastering high school algebra 1, 10 full length clep college algebra practice tests the practice you need to ace the clep college algebra test.

by: Effortless Math Team about 5 years ago (category: Articles , Free Math Worksheets )

What people say about "How to Solve Geometric Sequences? (+FREE Worksheet!) - Effortless Math: We Help Students Learn to LOVE Mathematics"?

No one replied yet.

Leave a Reply Cancel reply

You must be logged in to post a comment.

Pre-Algebra Practice Workbook The Most Comprehensive Review of Pre-Algebra

Algebra i practice workbook the most comprehensive review of algebra 1, algebra ii practice workbook the most comprehensive review of algebra 2, algebra i for beginners the ultimate step by step guide to acing algebra i, algebra ii for beginners the ultimate step by step guide to acing algebra ii, pre-algebra tutor everything you need to help achieve an excellent score.

• ATI TEAS 6 Math
• ISEE Upper Level Math
• SSAT Upper-Level Math
• Praxis Core Math
• 8th Grade STAAR Math

Limited time only!

Save Over 45 %

It was $89.99 now it is $49.99

Login and use all of our services.

Effortless Math services are waiting for you. login faster!

Register Fast!

Password will be generated automatically and sent to your email.

After registration you can change your password if you want.

• Math Worksheets
• Math Courses
• Math Topics
• Math Puzzles
• Math eBooks
• GED Math Books
• HiSET Math Books
• ACT Math Books
• ISEE Math Books
• ACCUPLACER Books
• Premium Membership
• Youtube Videos

Effortless Math provides unofficial test prep products for a variety of tests and exams. All trademarks are property of their respective trademark owners.

• Bulk Orders
• Refund Policy

Geometric Sequences

A series of free, online lessons for Intermediate Algebra (Algebra II) with videos, examples and solutions.

Related Pages Number Sequences Arithmetic Sequences Quadratic and Cubic Sequences More Lessons for Algebra II

In these lessons, we will learn

• about geometric sequences
• how to find the common ratio of a geometric sequence
• how to find the formula for the nth term of an geometric sequence
• how to find the sum of an geometric series

The following figure gives the formula for the nth term of a geometric sequence. Scroll down the page for more examples and solutions.

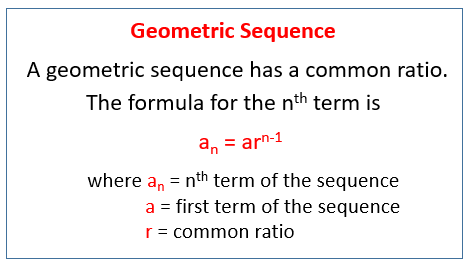
A geometric sequence is a sequence that has a pattern of multiplying by a constant to determine consecutive terms.

We say geometric sequences have a common ratio. The formula is a n = a n-1 r

A sequence is a function. What is the domain and range of the following sequence? What is r? -12, 6, -3, 3/2, -3/4

Given the formula for the geometric sequence, determine the first 2 terms and then the 5th term. Also state the common ratio.

Given the geometric sequence, determine the formula. Then determine the 6th term. 1/3, 2/9, 4/27, 8/81, …

A Quick Introduction To Geometric Sequences

This video gives the definition of a geometric sequence and go through 4 examples, determining if each qualifies as a geometric sequence or not.

A geometric sequence is a sequence of numbers where each term after the first term is found by multiplying the previous one by a fixed non-zero number, called the common ratio.

Example: Determine which of the following sequences are geometric. If so, give the value of the common ratio, r.

3,6,12,24,48,96, …

3,3/2,3/4,3/8,3/16,3/32,3/62, …

10,15,20,25,30, …

-1,.1,-.01,-.001,-.0001, …

A list of numbers that follows a rule is called a sequence. Sequences whose rule is the multiplication of a constant are called geometric sequences, similar to arithmetic sequences that follow a rule of addition. Homework problems on geometric sequences often ask us to find the nth term of a sequence using a formula. Geometric sequences are important to understanding geometric series.

How To Find The General Term Of A Geometric Sequence?

Example: Find the formula for the general term or nth term of a geometric sequence.

Geometric Sequences And Series

A short introduction to geometric sequences and series.

Math Skills & Equations: Solving Math Sequences

There are two kinds of math sequences that can be solved: arithmetic sequences and geometric sequences. An arithmetic sequence is solved by adding or subtracting the same number, while geometric sequences use division and multiplication. Learn more about solving math sequences.

Example: Find the 9th term of 3,12,48,192, …

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.