experimental probability definition example

Before you go, check this out!

We have lots more on the site to show you. You've only seen one page. Check out this post which is one of the most popular of all time.

Theoretical vs. Experimental Probability: How do they differ?

Probability is the study of chances and is an important topic in mathematics. There are two types of probability: theoretical and experimental.

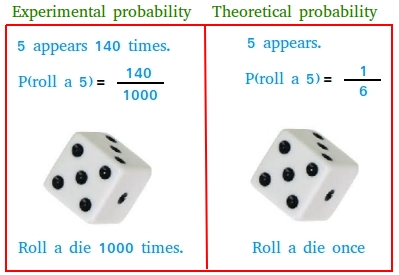
So, how to define theoretical and experimental probability? Theoretical probability is calculated using mathematical formulas, while experimental probability is based on results from experiments or surveys. In order words, theoretical probability represents how likely an event is to happen. On the other hand, experimental probability illustrates how frequently an event occurs in an experiment.

Read on to find out the differences between theoretical and experimental probability. If you wonder How to Understand Statistics Easily , I wrote a whole article where I share 9 helpful tips to help you Ace statistics.

Table of Contents

What Is Theoretical Probability?

Theoretical probability is calculated using mathematical formulas. In other words, a theoretical probability is a probability that is determined based on reasoning. It does not require any experiments to be conducted. Theoretical probability can be used to calculate the likelihood of an event occurring before it happens.

Keep in mind that theoretical probability doesn’t involve any experiments or surveys; instead, it relies on known information to calculate the chances of something happening.

For example, if you wanted to calculate the probability of flipping a coin and getting tails, you would use the formula for theoretical probability. You know that there are two possible outcomes—heads or tails—and that each outcome is equally likely, so you would calculate the probability as follows: 1/2, or 50%.

How Do You Calculate Theoretical Probability?

• First, start by counting the number of possible outcomes of the event.
• Second, count the number of desirable (favorable) outcomes of the event.
• Third, divide the number of desirable (favorable) outcomes by the number of possible outcomes.
• Finally, express this probability as a decimal or percentage.

The theoretical probability formula is defined as follows: Theoretical Probability = Number of favorable (desirable) outcomes divided by the Number of possible outcomes.

How Is Theoretical Probability Used in Real Life?

Probability plays a vital role in the day to day life. Here is how theoretical probability is used in real life: 

• Sports and gaming strategies
• Analyzing political strategies.
• Buying or selling insurance
• Determining blood groups 
• Online shopping
• Weather forecast
• Online games

What Is Experimental Probability?

Experimental probability, on the other hand, is based on results from experiments or surveys. It is the ratio of the number of successful trials divided by the total number of trials conducted. Experimental probability can be used to calculate the likelihood of an event occurring after it happens.

For example, if you flipped a coin 20 times and got heads eight times, the experimental probability of obtaining heads would be 8/20, which is the same as 2/5, 0.4, or 40%.

How Do You Calculate Experimental Probability?

The formula for the experimental probability is as follows:  Probability of an Event P(E) = Number of times an event happens divided by the Total Number of trials .

If you are interested in learning how to calculate experimental probability, I encourage you to watch the video below.

How Is Experimental Probability Used in Real Life?

Knowing experimental probability in real life provides powerful insights into probability’s nature. Here are a few examples of how experimental probability is used in real life:

• Rolling dice
• Selecting playing cards from a deck
• Drawing marbles from a hat
• Tossing coins

The main difference between theoretical and experimental probability is that theoretical probability expresses how likely an event is to occur, while experimental probability characterizes how frequently an event occurs in an experiment.

In general, the theoretical probability is more reliable than experimental because it doesn’t rely on a limited sample size; however, experimental probability can still give you a good idea of the chances of something happening.

The reason is that the theoretical probability of an event will invariably be the same, whereas the experimental probability is typically affected by chance; therefore, it can be different for different experiments.

Also, generally, the more trials you carry out, the more times you flip a coin, and the closer the experimental probability is likely to be to its theoretical probability.

Also, note that theoretical probability is calculated using mathematical formulas, while experimental probability is found by conducting experiments.

What to read next:

• Types of Statistics in Mathematics And Their Applications .
• Is Statistics Harder Than Algebra? (Let’s find out!)
• Should You Take Statistics or Calculus in High School?
• Is Statistics Hard in High School? (Yes, here’s why!)

Wrapping Up

Theoretical and experimental probabilities are two ways of calculating the likelihood of an event occurring. Theoretical probability uses mathematical formulas, while experimental probability uses data from experiments. Both types of probability are useful in different situations.

I believe that both theoretical and experimental probabilities are important in mathematics. Theoretical probability uses mathematical formulas to calculate chances, while experimental probability relies on results from experiments or surveys.

I am Altiné. I am the guy behind mathodics.com. When I am not teaching math, you can find me reading, running, biking, or doing anything that allows me to enjoy nature's beauty. I hope you find what you are looking for while visiting mathodics.com.

Recent Posts

How to Find the Y-Value of Stationary Points with TI-84 Plus CE

TI-84 Plus CE Calculator If you’re studying calculus or any advanced math course, you will certainly come across the concept of stationary points. So, what is a stationary point? A...

IB Maths Vs. A-Level Maths - Which One is Harder?

Maths is a subject that can be demanding for many students. It not only requires strong analytical skills but also an ability to handle complex concepts with ease. Students looking to further their...

Experimental Probability

The chance or occurrence of a particular event is termed its probability. The value of a probability lies between 0 and 1 which means if it is an impossible event, the probability is 0 and if it is a certain event, the probability is 1. The probability that is determined on the basis of the results of an experiment is known as experimental probability. This is also known as empirical probability.

What is Experimental Probability?

Experimental probability is a probability that is determined on the basis of a series of experiments. A random experiment is done and is repeated many times to determine their likelihood and each repetition is known as a trial. The experiment is conducted to find the chance of an event to occur or not to occur. It can be tossing a coin, rolling a die, or rotating a spinner. In mathematical terms, the probability of an event is equal to the number of times an event occurred ÷ the total number of trials. For instance, you flip a coin 30 times and record whether you get a head or a tail. The experimental probability of obtaining a head is calculated as a fraction of the number of recorded heads and the total number of tosses. P(head) = Number of heads recorded ÷ 30 tosses.

Experimental Probability Formula

The experimental probability of an event is based on the number of times the event has occurred during the experiment and the total number of times the experiment was conducted. Each possible outcome is uncertain and the set of all the possible outcomes is called the sample space. The formula to calculate the experimental probability is: P(E) = Number of times an event occurs/Total number of times the experiment is conducted

Consider an experiment of rotating a spinner 50 times. The table given below shows the results of the experiment conducted. Let us find the experimental probability of spinning the color - blue.

The experimental probability of spinning the color blue = 10/50 = 1/5 = 0.2 = 20%

Experimental Probability vs Theoretical Probability

Experimental results are unpredictable and may not necessarily match the theoretical results. The results of experimental probability are close to theoretical only if the number of trials is more in number. Let us see the difference between experimental probability and theoretical probability.

Experimental Probability Examples

Here are a few examples from real-life scenarios.

a) The number of cookies made by Patrick per day in this week is given as 4, 7, 6, 9, 5, 9, 5.

Based on this data, what is the reasonable estimate of the probability that Patrick makes less than 6 cookies the next day?

P(< 6 cookies) = 3/7 = 0.428 = 42%

b) Find the reasonable estimate of the probability that while ordering a pizza, the next order will not be of a pepperoni topping.

Based on this data , the reasonable estimate of the probability that the next type of toppings that would get ordered is not a pepperoni will be 15/20 = 3/4 = 75%

Related Sections

• Card Probability
• Conditional Probability Calculator
• Binomial Probability Calculator
• Probability Rules
• Probability and Statistics

Important Notes

• The sum of the experimental probabilities of all the outcomes is 1.
• The probability of an event lies between 0 and 1, where 0 is an impossible event and 1 denotes a certain event.
• Probability can also be expressed in percentage.

Examples on Experimental Probability

Example 1: The following table shows the recording of the outcomes on throwing a 6-sided die 100 times.

Find the experimental probability of: a) Rolling a four; b) Rolling a number less than four; c) Rolling a 2 or 5

Experimental probability is calculated by the formula: Number of times an event occurs/Total number of trials

a) Rolling a 4: 17/100 = 0.17

b) Rolling a number less than 4: 56/100 = 0.56

c) Rolling a 2 or 5: 31/100 = 0.31

Example 2: The following set of data shows the number of messages that Mike received recently from 6 of his friends. 4, 3, 2, 1, 6, 8. Based on this, find the probability that Mike will receive less than 2 messages next time.

Mike has received less than 2 messages from 2 of his friends out of 6.

Therefore, P(<2) = 2/6 = 1/3

go to slide go to slide

Book a Free Trial Class

Practice Questions on Experimental Probability

Frequently asked questions (faqs), how do you find the experimental probability.

The experimental probability of an event is based on actual experiments and the recordings of the events. It is equal to the number of times an event occurred divided by the total number of trials.

What is the Experimental Probability of rolling a 6?

The experimental probability of rolling a 6 is 1/6. A die has 6 faces numbered from 1 to 6. Rolling the die to get any number from 1 to 6 is the same and the probability (of getting a 6) = Number of favorable outcomes/ total possible outcomes = 1/6.

What is the Difference Between Theoretical and Experimental Probability?

Theoretical probability is what is expected to happen and experimental probability is what has actually happened in the experiment.

Do You Simplify Experimental Probability?

Yes, after finding the ratio of the number of times the event occurred to the total number of trials conducted, the fraction which is obtained is simplified.

Which Probability is More Accurate, Theoretical Probability or Experimental Probability?

Theoretical probability is more accurate than experimental probability. The results of experimental probability are close to theoretical only if the number of trials are more in number.

• Member Login
• Pre-Algebra Lessons
• Pre-Algebra Word Problems
• Pre-Algebra Calculators
• Algebra Lessons
• Algebra Word Problems
• Algebra Proofs
• Advanced Algebra
• Algebra Calculators
• Geometry Lessons
• Geometry Word Problems
• Geometry Proofs
• Geometry Calculators
• Trigonometry Lessons
• Numeration System
• Basic Concepts of Set Theory
• Consumer Math
• Baseball Math
• Math for Nurses
• Statistics Made Easy
• High School Physics
• Basic Mathematics Store
• Math Vocabulary Quizzes
• SAT Math Prep
• Math Skills by Grade Level
• Ask an Expert
• Other Websites
• K-12 Worksheets
• Worksheets Generator
• Algebra Worksheets
• Geometry Worksheets
• Math Puzzles
• Math Tricks

Experimental probability

Experimental probability (EP), also called empirical probability or relative frequency , is probability based on data collected from repeated trials.

Experimental probability formula

Let n represent the total number of trials or the number of times an experiment is done. Let p represent the number of times an event occurred while performing this experiment n times.

Example #1: A manufacturer makes 50,000 cell phones every month. After inspecting 1000 phones, the manufacturer found that 20 phones are defective. What is the probability that you will buy a phone that is defective? Predict how many phones will be defective next month.

The total number of times the experiment is conducted is n = 1000

The number of times an event occurred is p  = 20 

Experimental probability is performed when authorities want to know how the public feels about a matter. Since it is not possible to ask every single person in the country, they may conduct a survey by asking a sample of the entire population. This is called population sampling. Example #2 is an example of this situation.

There are about 319 million people living in the USA. Pretend that a survey of 1 million people revealed that 300,000 people think that all cars should be electric. What is the probability that someone chosen randomly does not like electric car? How many people like electric cars?

Notice that the number of people who do not like electric cars is 1000000 - 300000 = 700000

Difference between experimental probability and theoretical probability

You can argue the same thing using a die, a coin, and a spinner. We will though use a coin and a spinner to help you see the difference.

Using a coin 

In theoretical probability, we say that "each outcome is equally likely " without the actual experiment. For instance, without  flipping a coin, you know that the outcome could either be heads or tails.  If the coin is not altered, we argue that each outcome (heads or tails) is equally likely. In other words, we are saying that in theory or (supposition, conjecture, speculation, assumption, educated guess) the probability to get heads is 50% or the probability to get tails in 50%. Since you did not actually flip the coin, you are making an assumption based on logic.

The logic is that there are 2 possible outcomes and since you are choosing 1 of the 2 outcomes, the probability is 1/2 or 50%. This is theoretical probability or guessing probability or probability based on assumption.

In the example above about flipping a coin, suppose you are looking for the probability to get a head. 

Then, the number of favorable outcomes is 1 and the number of possible outcomes is 2.

In experimental probability,  we want to take the guess work out of the picture, by doing the experiment to see how many times heads or teals will come up. If you flip a coin 1000 times, you might realize that it landed on heads only 400 times. In this case, the probability to get heads is only 40%. 

Your experiment may not even show tails until after the 4th flip and yet in the end you ended up with more tails than heads. 

If you repeat the experiment another day, you may find a completely different result. May be this time the number of heads is 600 and the number of tails is 400.

Using a spinner

Suppose a spinner has four equal-sized sections that are red, green, black, and yellow. 

In theoretical probability, you will not spin the spinner. Instead, you will say that the probability to get green is one-fourth or 25%. Why 25%? The total number of outcomes is 4 and the number of favorable outcomes is 1.

1/4 = 0.25 = 25%

However, in experimental probability, you may decide to spin the spinner 50 times or even more to see how many times you will get each color.

Suppose you spin the spinner 50 times. It is quite possible that you may end up with the result shown below:

Red: 10 Green: 15 Black: 5 Yellow: 20

Now, the probability to get green is 15/50 = 0.3 = 30%

As you can see, experimental probability is based more on facts, data collected, experiment or research!

Theoretical probability

Special Math Topics

Applied math.

About me :: Privacy policy :: Disclaimer :: Donate   Careers in mathematics  

Copyright © 2008-2021. Basic-mathematics.com. All right reserved

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

In order to access this I need to be confident with:

Prob. distribution

Experimental prob.

Experimental probability

Here you will learn about experimental probability, including using the relative frequency and finding the probability distribution.

Students will first learn about experimental probability as part of statistics and probability in 7 th grade.

What is experimental probability?

Experimental probability is the probability of an event happening based on an experiment or observation.

To calculate the experimental probability of an event, you calculate the relative frequency of the event.

Relative frequency =\cfrac{\text{frequency of event occurring}}{\text{total number of trials of the experiment}}

You can also express this as R=\cfrac{f}{n} where R is the relative frequency, f is the frequency of the event occurring, and n is the total number of trials of the experiment.

If you find the relative frequency for all possible events from the experiment, you can write the probability distribution for that experiment.

The relative frequency, experimental probability, and empirical probability are the same thing and are calculated using the data from random experiments. They also have a key use in real-life problem-solving.

For example, Jo made a four-sided spinner out of cardboard and a pencil.

She spun the spinner 50 times. The table shows the number of times the spinner landed on each of the numbers 1 to 4. The final column shows the relative frequency.

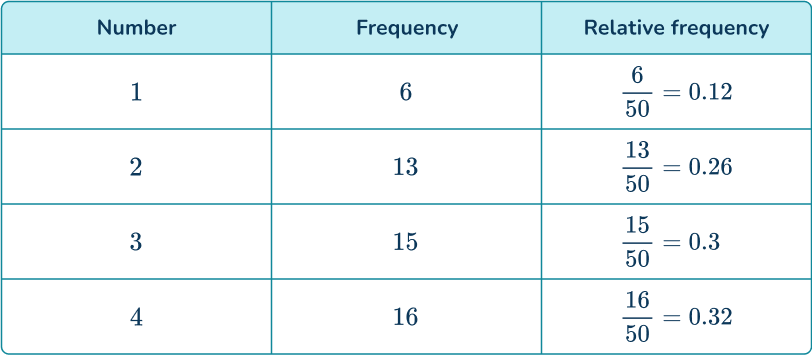
The relative frequencies of all possible events will add up to 1.

This is because the events are mutually exclusive.

See also: Mutually exclusive events

[FREE] Probability Check for Understanding Quiz (Grade 7 to 12)

Use this quiz to check your grade 7 to 12 students’ understanding of probability. 15+ questions with answers covering a range of 7th to 12th grade probability topics to identify areas of strength and support!

Experimental probability vs theoretical probability

You can see that the relative frequencies are not equal to the theoretical probabilities you would expect if the spinner was fair.

If the spinner is fair, the more times an experiment is done, the closer the relative frequencies should be to the theoretical probabilities.

In this case, the theoretical probability of each section of the spinner would be 0.25, or \cfrac{1}{4}.

Step-by-step guide: Theoretical probability

Common Core State Standards

How does this relate to 7 th grade math?

• Grade 7 – Statistics & Probability (7.SP.C.5) Understand that the probability of a chance event is a number between 0 and 1 that expresses the likelihood of the event occurring. Larger numbers indicate greater likelihood. A probability near 0 indicates an unlikely event, a probability around \cfrac{1}{2} indicates an event that is neither unlikely nor likely, and a probability near 1 indicates a likely event.

How to find an experimental probability distribution

In order to calculate an experimental probability distribution:

Draw a table showing the frequency of each outcome in the experiment.

Determine the total number of trials.

Write the experimental probability (relative frequency) of the required outcome(s).

Experimental probability examples

Example 1: finding an experimental probability distribution.

A 3- sided spinner numbered 1, \, 2, and 3 is spun and the results are recorded.

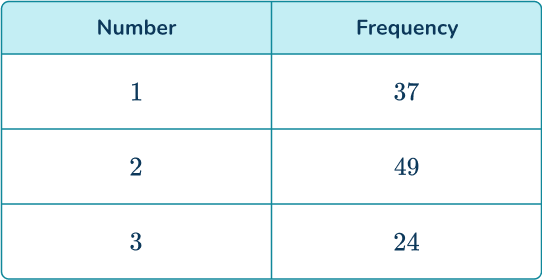
Find the probability distribution for the 3- sided spinner from these experimental results.

A table of results has already been provided. You can add an extra column for the relative frequencies.

2 Determine the total number of trials.

3 Write the experimental probability (relative frequency) of the required outcome(s).

Divide each frequency by 110 to find the relative frequencies.

Example 2: finding an experimental probability distribution

A normal 6- sided die is rolled 50 times. A tally chart was used to record the results.

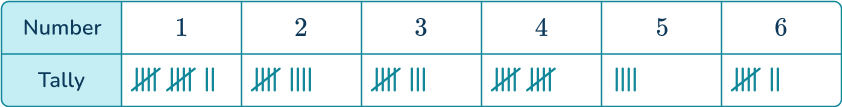
Determine the probability distribution for the 6- sided die. Give your answers as decimals.

Use the tally chart to find the frequencies and add a row for the relative frequencies.

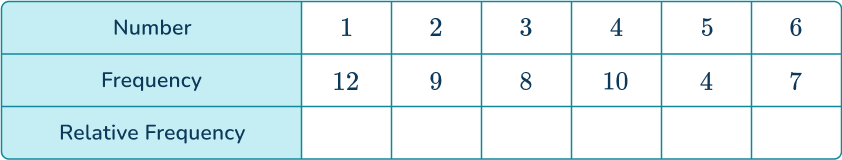
The question stated that the experiment had 50 trials. You can also check that the frequencies add up to 50.

Divide each frequency by 50 to find the relative frequencies.

Example 3: using an experimental probability distribution

A student made a biased die and wanted to find its probability distribution for use in a game. They rolled the die 100 times and recorded the results.

By calculating the probability distribution for the die, determine the probability of the die landing on a 3 or a 4.

The die was rolled 100 times.

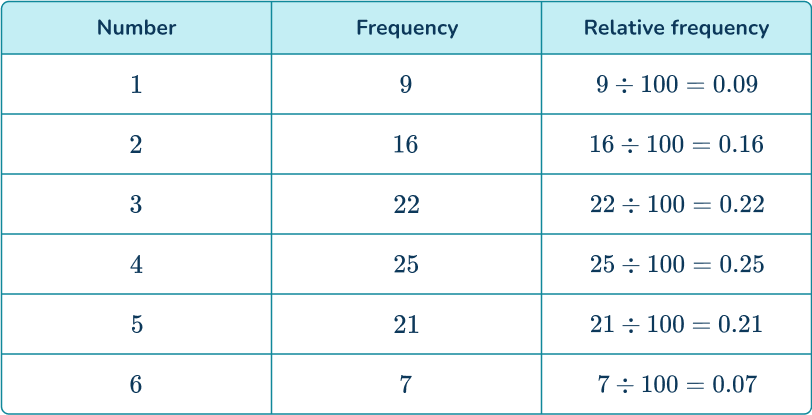
You can find the probability of rolling a 3 or a 4 by adding the relative frequencies for those numbers.

P(3\text{ or }4)=0.22+0.25=0.47

Note: P(\text{Event }A) means the probability of event A occurring.

Alternatively, it is only necessary to calculate the relative frequencies for the desired events but by calculating all of the relative frequencies and finding the sum of these values, your solution should equal 1.

The frequency of rolling a 3 or a 4 is 22+25=47.

As the total frequency is 100, the relative frequency is \cfrac{47}{100}=0.47.

Example 4: calculating the relative frequency without a known frequency of outcomes

A research study asked 1,200 people how they commute to work. 640 travel by car, 174 use the bus, and the rest walk. Determine the relative frequency of someone walking to work.

Writing the known information into a table, you have

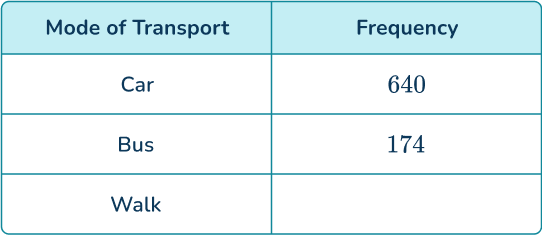
You currently do not know the frequency of people who walk to work. You can calculate this as you know the total frequency.

The number of people who walk to work is equal to

1200-(640+174)=386.

You now have the full table,

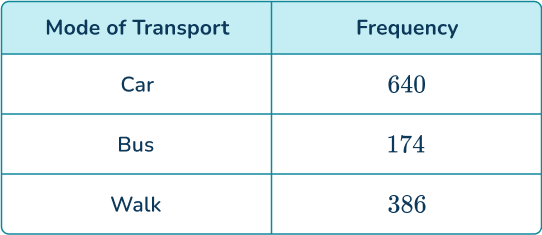
The total frequency is 1,200.

Divide each frequency by the total number of people (1,200), you have

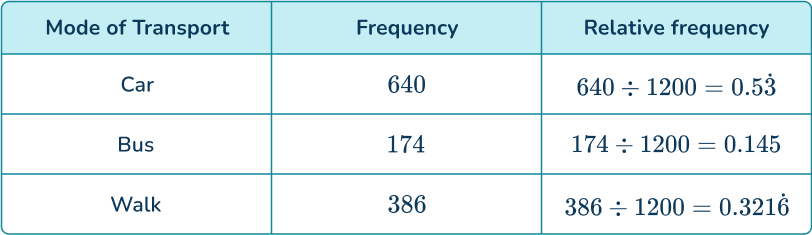
The relative frequency of someone walking to work is 0.3216.

How to find a frequency using an experimental probability

In order to calculate a frequency using an experimental probability:

Determine the experimental probability of the event.

Multiply the total frequency by the experimental probability.

Example 5: calculating a frequency

A dice was rolled 300 times. The experimental probability of rolling an even number is \cfrac{27}{50}. How many times was an even number rolled?

The experimental probability is \cfrac{27}{50}.

An even number was rolled 162 times.

Example 6: calculating a frequency

A bag contains different colored counters. A counter is selected at random and replaced back into the bag 240 times. The probability distribution of the experiment is given below.

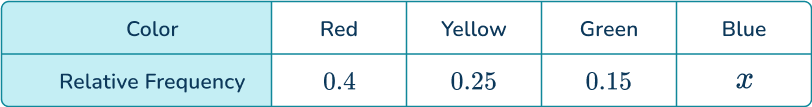
Determine the total number of times a blue counter was selected.

As the events are mutually exclusive, the sum of the probabilities must be equal to 1.

This means that you can determine the value of x.

1-(0.4+0.25+0.15)=0.2

The experimental probability (relative frequency) of a blue counter is 0.2.

Multiplying the total frequency by 0.2, you have

240 \times 0.2=48

A blue counter was selected 48 times.

Teaching tips for experimental probability

• Relate probability to everyday situations, such as the chance of getting heads or tails when flipping a fair coin, to make the concept more tangible.
• Rather than strictly using worksheets, let students conduct their own experiments, such as rolling dice or drawing marbles from a bag, to collect data and compute probabilities.
• Emphasize that in mathematics, experimental probability is based on actual trials or experiments, as opposed to theoretical probability which is based on possible outcomes.
• Teach students how to record the results of an experiment systematically and use them to calculate probabilities. Use charts or tables to help visualize the data.
• Discuss events that cannot occur, such as rolling a 7 with a single six-sided die. Explain that the probability of impossible events is always 0. This helps students understand the concept of probability in a broader context.

Easy mistakes to make

• Forgetting the differences between theoretical and experimental probability It is common to forget to use the relative frequencies from experiments for probability questions and use the theoretical probabilities instead. For example, they may be asked to find the probability of a die landing on an even number based on an experiment and the student will incorrectly answer it as 0.5.
• Thinking the relative frequency is an integer The relative frequency is the same as the experimental probability. This value is written as a fraction, decimal, or percentage, not an integer.
• Assuming future results will be the same Students might think that if an experiment yields a certain probability on one day, the results will be the same the next day. Explain that while probabilities are consistent over time in theory, each set of trials can have different outcomes due to randomness, and variations can occur from day to day.

Related probability distribution lessons

• Probability distribution
• Expected frequency

Practice experimental probability questions

1. A coin is flipped 80 times and the results are recorded.

Determine the probability distribution of the coin.

As the number of tosses is 80, dividing the frequencies for the number of heads and the number of tails by 80, you have

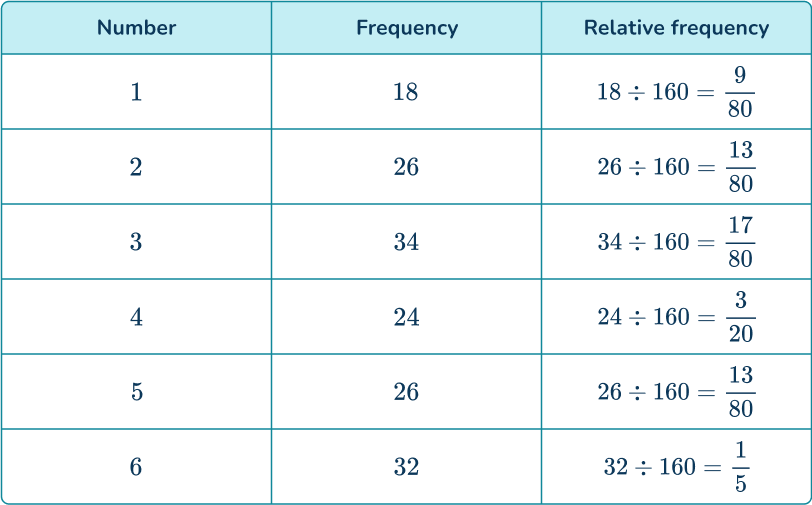
2. A 6- sided die is rolled 160 times and the results are recorded.

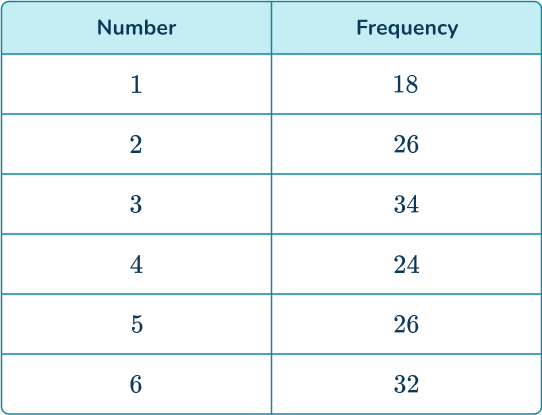
Determine the probability distribution of the die. Write your answers as fractions in their simplest form.

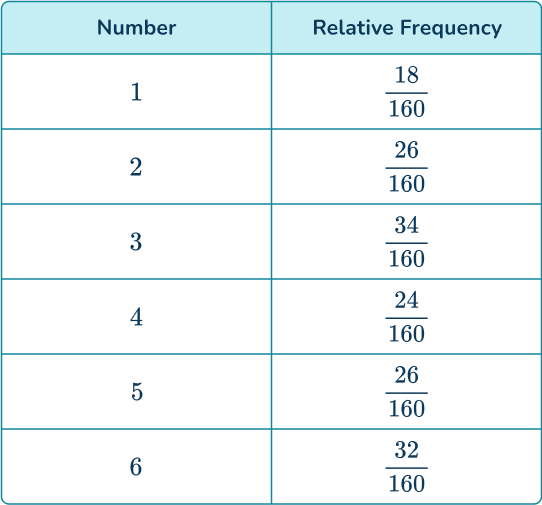
Dividing the frequencies of each number by 160, you get

3. A 3- sided spinner is spun and the results are recorded.

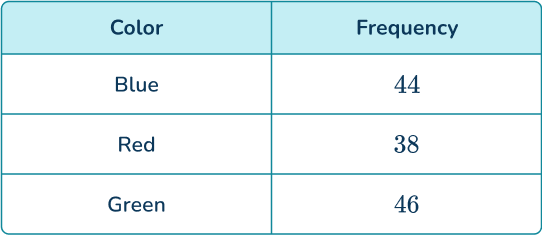
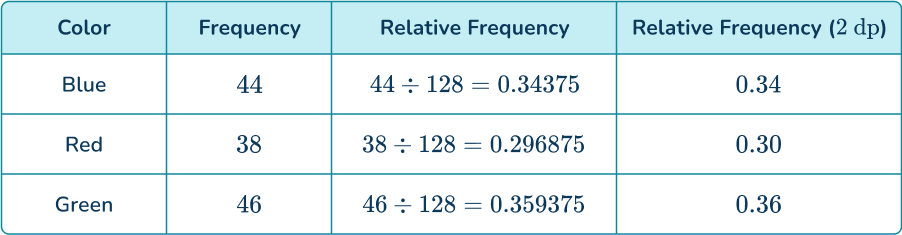
Find the probability distribution of the spinner, giving your answers as decimals to 2 decimal places.

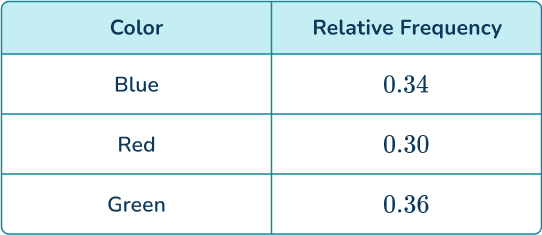
By dividing the frequencies of each color by 128 and simplifying, you have

4. A 3- sided spinner is spun and the results are recorded.

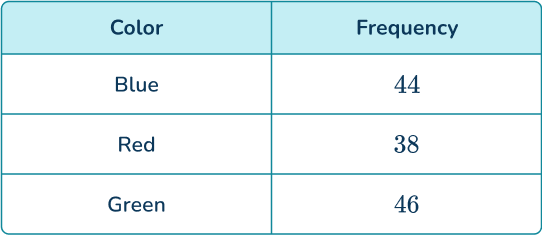
Find the probability of the spinner not landing on red. Give your answer as a fraction.

Add the frequencies of blue and green and divide by 128.

5. A card is picked at random from a deck and then replaced. This was repeated 4,000 times. The probability distribution of the experiment is given below.

How many times was a club picked?

6. Find the missing frequency from the probability distribution.

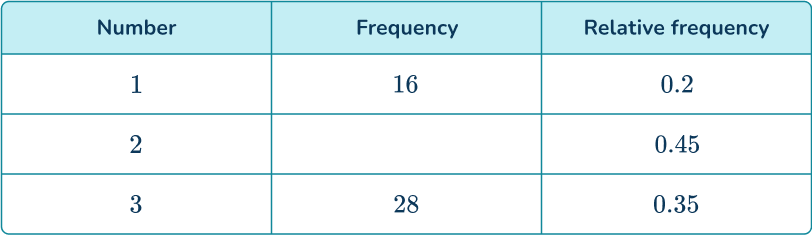
The total frequency is calculated by dividing the frequency by the relative frequency.

Experimental probability FAQs

Experimental probability is the likelihood of an event occurring based on the results of an actual experiment or trial. It is calculated as the ratio of the number of favorable outcomes to the total number of trials.

To calculate experimental probability, you calculate the relative frequency of the event: \text{Relative frequency}=\frac{\text{Frequency of event occurring}}{\text{Total number of trials of the experiment}}

Experimental Probability is based on actual results from an experiment or trial. Theoretical Probability is based on the possible outcomes of an event, calculated using probability rules and formulas without conducting experiments.

It helps us understand how likely events are in real-world scenarios based on actual data. For example, it can be used to predict outcomes in various fields such as social science, medicine, finance, and engineering.

The next lessons are

• Units of measurement
• Represent and interpret data

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs .

[FREE] Common Core Practice Tests (3rd to 8th Grade)

Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.

Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!

Privacy Overview

• Mathematicians
• Math Lessons
• Square Roots
• Math Calculators
• Experimental Probability – Explanation & Examples

JUMP TO TOPIC

What is experimental probability? 

Practice questions, experimental probability – explanation & examples.

Experimental probability is the probability determined based on the results from performing the particular experiment. 

In this lesson we will go through:

• The meaning of experimental probability
• How to find experimental probability

The ratio of the number of outcomes favorable to an event to the total number of trials of the experiment.

Experimental Probability can be expressed mathematically as: 

$P(\text{E}) = \frac{\text{number of outcomes favorable to an event}}{\text{total number of trials of the experiment}}$

Let’s go back to the die tossing example. If after 12 throws you get one 6, then the experimental probability is $\frac{1}{12}$.  You can compare that to the theoretical probability. The theoretical probability of getting a 6 is $\frac{1}{6}$. This means that in 12 throws we would have expected to get 6 twice. 

Similarly, if in those 12 tosses you got a 1 five times, the experimental probability is $\frac{5}{12}$. 

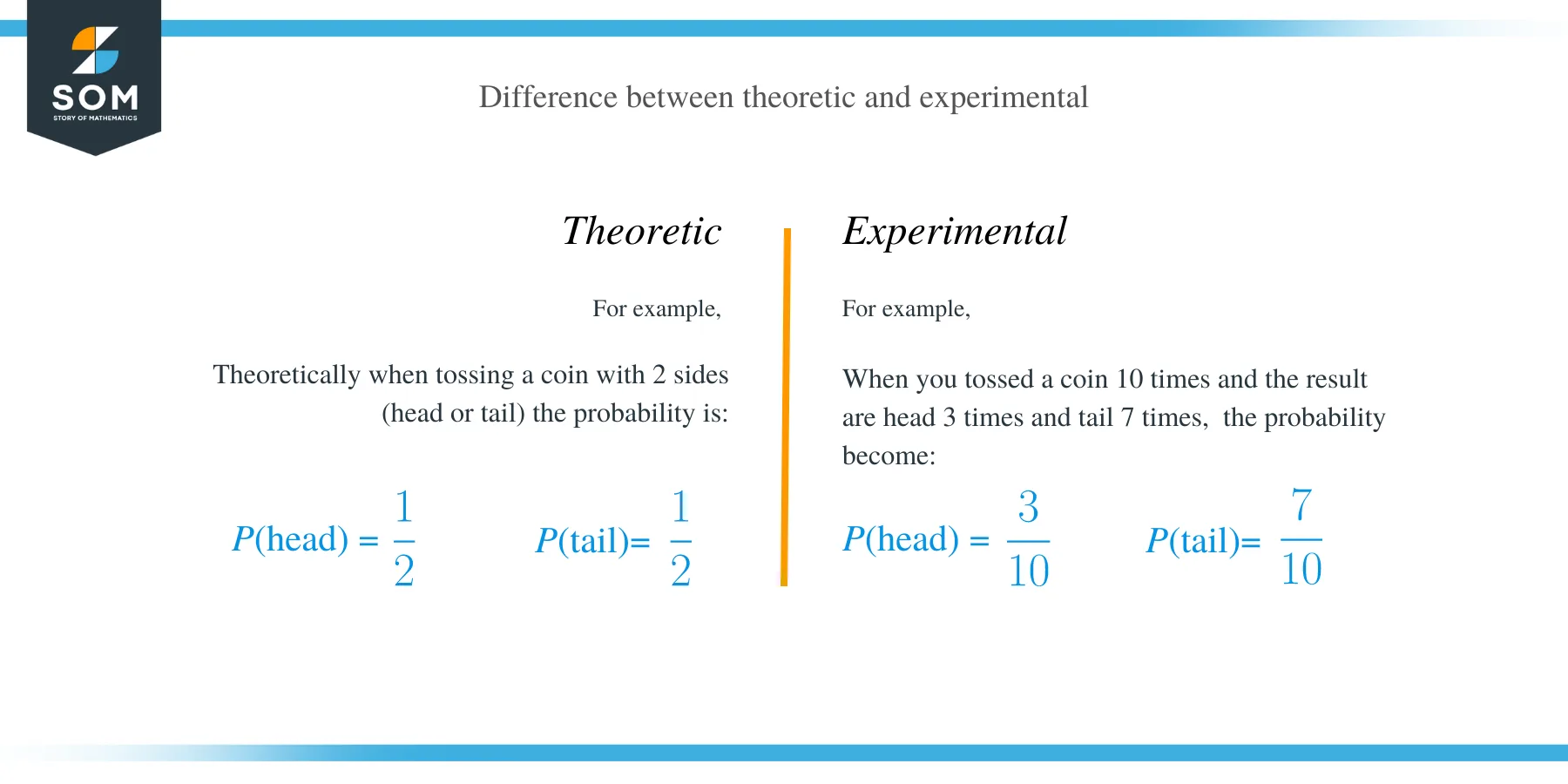
How do we find experimental probability?

Now that we understand what is meant by experimental probability, let’s go through how it is found. 

To find the experimental probability of an event, divide the number of observed outcomes favorable to the event by the total number of trials of the experiment. 

Let’s go through some examples. 

Example 1:  There are 20 students in a class. Each student simultaneously flipped one coin. 12 students got a Head. From this experiment, what was the experimental probability of getting a head?

Number of coins showing Heads: 12

Total number of coins flipped: 20

$P(\text{Head}) = \frac{12}{20} = \frac{3}{5}$ 

Example 2:  The tally chart below shows the number of times a number was shown on the face of a tossed die. 

a. What was the probability of a 3 in this experiment?

b. What was the probability of a prime number?

First, sum the numbers in the frequency column to see that the experiment was performed 30 times. Then find the probabilities of the specified events. 

a. Number of times 3 showed = 7

Number of tosses = 30

$P(\text{3}) = \frac{7}{30}$ 

b. Frequency of primes = 6 + 7 + 2 = 15

Number of trials = 30 

$P(\text{prime}) = \frac{15}{30} = \frac{1}{2}$

Experimental probability can be used to predict the outcomes of experiments. This is shown in the following examples. 

Example 3: The table shows the attendance schedule of an employee for the month of May.

a. What is the probability that the employee is absent? 

b. How many times would we expect the employee to be present in June?

a. The employee was absent three times and the number of days in this experiment was 31. Therefore:

$P(\text{Absent}) = \frac{3}{31}$

b.  We expect the employee to be absent

$\frac{3}{31} × 30 = 2.9 ≈ 3$ days in June 

Example 4:  Tommy observed the color of cars owned by people in his small hometown. Of the 500 cars in town, 10 were custom colors, 100 were white, 50 were red, 120 were black, 100 were silver, 60 were blue, and 60 were grey. 

a. What is the probability that a car is red?

b. If a new car is bought by someone in town, what color do you think it would be? Explain. 

a. Number of red cars = 50 

Total number of cars = 500 

$P(\text{red car}) = \frac{50}{500} = \frac{1}{10}$ 

b. Based on the information provided, it is most likely that the new car will be black. This is because it has the highest frequency and the highest experimental probability. 

Now it is time for you to try these examples. 

The table below shows the colors of jeans in a clothing store and their respective frequencies. Use the table to answer the questions that follow.

• What is the probability of selecting a brown jeans?
• What is the probability of selecting a blue or a white jeans?

On a given day, a fast food restaurant notices that it sold 110 beef burgers, 60 chicken sandwiches, and 30 turkey sandwiches. From this observation, what is the experimental probability that a customer buys a beef burger?

Over a span of 20 seasons, a talent competition notices the following. Singers won 12 seasons, dancers won 2 seasons, comedians won 3 seasons, a poet won 1 season, and daring acts won the other 2 seasons. 

a. What is the experimental probability of a comedian winning  a season?

b. From the next 10 seasons, how many winners do you expect to be dancers?

Try this at home! Flip a coin 10 times. Record the number of tails you get. What is your P(tail)?

Number of brown jeans = 25

Total Number of jeans = 125

$P(\text{brown}) = \frac{25}{125}  = \frac{1}{5}$

Number of jeans that are blue or white = 75 + 20 = 95

$P(\text{blue or white}) = \frac{95}{125} = \frac{19}{25}$

Number of beef burgers = 110 

Number of burgers (or sandwiches) sold = 200 

$P(\text{beef burger}) = \frac{110}{200} = \frac{11}{20}$ 

a. Number of comedian winners = 3

Number of seasons = 20 

$P(\text{comedian}) = \frac{3}{20}$ 

b. First find the experimental probability that the winner is a dancer. 

Number of winners that are dancers = 2 

$P(\text{dancer}) = \frac{2}{20} = \frac{1}{10}$ 

Therefore we expect 

$\frac{1}{10} × 10 = 1$ winner to be a dancer in the next 10 seasons.

To find your P(tail) in 10 trials, complete the following with the number of tails you got. 

$P(\text{tail}) = \frac{\text{number of tails}}{10}$ 

Previous Lesson  |  Main Page | Next Lesson

• Math Article
• Experimental Probability

You and your 3 friends are playing a board game. It’s your turn to roll the die and to win the game you need a 5 on the dice. Now, is it possible that upon rolling the die you will get an exact 5? No, it is a matter of chance. We face multiple situations in real life where we have to take a chance or risk. Based on certain conditions, the chance of occurrence of a certain event can be easily predicted. In our day to day life, we are more familiar with the word ‘ chance and probability ’. In simple words, the chance of occurrence of a particular event is what we study in probability. In this article, we are going to discuss one of the types of probability called  “Experimental Probability” in detail.

What is Probability?

Probability, a branch of Math that deals with the likelihood of the occurrences of the given event. The probability values for the given experiment is usually defined between the range of numbers. The values lie between the numbers 0 and 1. The probability value cannot be a negative value. The basic rules such as addition, multiplication and complement rules are associated with the probability.

Experimental Probability Vs Theoretical Probability

There are two approaches to study probability:

• Theoretical Probability

What is Experimental Probability?

Experimental probability, also known as Empirical probability, is based on actual experiments and adequate recordings of the happening of events. To determine the occurrence of any event, a series of actual experiments are conducted. Experiments which do not have a fixed result are known as random experiments. The outcome of such experiments is uncertain. Random experiments are repeated multiple times to determine their likelihood. An experiment is repeated a fixed number of times and each repetition is known as a trial. Mathematically, the formula for the experimental probability is defined by;

Probability of an Event P(E) = Number of times an event occurs / Total number of trials.

What is Theoretical Probability?

In probability, the theoretical probability is used to find the probability of an event. Theoretical probability does not require any experiments to conduct. Instead of that, we should know about the situation to find the probability of an event occurring. Mathematically, the theoretical probability is described as the number of favourable outcomes divided by the number of possible outcomes.

Probability of Event P(E) = No. of. Favourable outcomes/ No. of. Possible outcomes.

Experimental Probability Example

Example: You asked your 3 friends Shakshi, Shreya and Ravi to toss a fair coin 15 times each in a row and the outcome of this experiment is given as below:

Calculate the probability of occurrence of heads and tails.

Solution: The experimental probability for the occurrence of heads and tails in this experiment can be calculated as:

Experimental Probability of Occurrence of heads = Number of times head occurs/Number of times coin is tossed.

Experimental Probability of Occurrence of tails = Number of times tails occurs/Number of times coin is tossed.

We observe that if the number of tosses of the coin increases then the probability of occurrence of heads or tails also approaches to 0.5.

To know more about experimental probability and theoretical probability please download BYJU’S – The Learning App.

Leave a Comment Cancel reply

Your Mobile number and Email id will not be published. Required fields are marked *

Request OTP on Voice Call

Post My Comment

Register with BYJU'S & Download Free PDFs

Register with byju's & watch live videos.

• Maths Notes Class 9
• NCERT Solutions Class 9
• RD Sharma Solutions Class 9
• Maths Formulas Class 9
• Class 9 Syllabus
• Class 9 Revision Notes
• Physics Notes Class 9
• Chemistry Notes Class 9
• Biology Notes Class 9
• History Notes class 9
• Geography Notes class 9
• Social science Notes class 9
• Experimental Probability

Experimental probability , also known as empirical probability , is a concept in mathematics that deals with estimating the likelihood of an event occurring based on actual experimental results. Unlike theoretical probability , which predicts outcomes based on known possibilities, experimental probability is derived from real-life experiments and observations.

To understand this better, imagine flipping a coin. The theoretical probability of landing heads is 50% or 1/2. However, if you actually flip the coin 100 times and record the outcomes, you might get heads 48 times. The experimental probability of getting heads would then be 48/100 or 0.48.

In this article, we will explore the concept of experimental probability, its significance, and how it differs from theoretical probability. We will discuss the formula for calculating experimental probability, provide examples to illustrate its application.

Table of Content

What is Probability?

What is experimental probability, formula for experimental probability, examples of experimental probability, what is theoretical probability, experimental probability vs theoretical probability.

• Solved Examples
• Practice Problems

The branch of mathematics that tells us about the likelihood of the occurrence of any event is the probability . Probability tells us about the chances of happening an event.

The probability of any element that is sure to occur is One(1) whereas the probability of any impossible event is Zero(0). The probability of all the elements ranges between 0 to 1.

There are two ways of studying probability that are

• Theoretical Probability

Now let’s learn about both in detail.

Experimental probability is a type of probability that is calculated by conducting an actual experiment or by performing a series of trials to observe the occurrence of an event. It is also known as empirical probability.

To calculate experimental probability, you need to conduct an experiment by repeating the event multiple times and observing the outcomes. Then, you can find the probability of the event occurring by dividing the number of times the event occurred by the total number of trials.

The experimental Probability for Event A can be calculated as follows:

P(E) = (Number of times an event occur in an experiment) / (Total number of Trials)

Now, as we learn the formula, let’s put this formula in our coin-tossing case.  If we tossed a coin 10 times and recorded a head 4 times and a tail 6 times then the Probability of Occurrence of Head on tossing a coin:

P(H) = 4/10

Similarly, the Probability of Occurrence of Tails on tossing a coin:

P(T) = 6/10

Theoretical Probability deals with assumptions in order to avoid unfeasible or expensive repetition experiments. The theoretical Probability for an Event A can be calculated as follows:

P(A) = Number of outcomes favorable to Event A / Number of all possible outcomes            

Now, as we learn the formula, let’s put this formula in our coin-tossing case. In tossing a coin, there are two outcomes: Head or Tail.

Hence, The Probability of occurrence of Head on tossing a coin is

Similarly, The Probability of the occurrence of a Tail on tossing a coin is

There are some key differences between Experimental and Theoretical Probability , some of which are as follows:

• Probability in Maths
• Probability Distribution
• Bayes’ Theorem

Solved Examples of Experimental Probability

Example 1. Let’s take an example of tossing a coin, tossing it 40 times , and recording the observations. By using the formula, we can find the experimental probability for heads and tails as shown in the below table.

Number of Trail Outcome Number of Trail Outcome Number of Trail Outcome Number of Trail Outcome First H Eleventh T Twenty-first T Thirty-first T Second T Twelfth T Twenty-second H Thirty-second H Third T Thirteenth H Twenty-third T Thirty-third T Fourth H Fourteenth H Twenty-fourth H Thirty-fourth H Fifth H Fifteenth H Twenty-fifth T Thirty-fifth T Sixth H Sixteenth H Twenty-sixth H Thirty-sixth T Seventh T Seventeenth T Twenty-seventh T Thirty-seventh T Eighth H Eighteenth T Twenty-eighth T Thirty-eighth H Ninth T Nineteenth T Twenty-ninth T Thirty-ninth T Tenth H Twentieth T Thirtieth H Fortieth T The formula for experimental probability: P(H) = Number of Heads ÷ Total Number of Trials = 16 ÷ 40 = 0.4 Similarly, P(H) = Number of Tails ÷ Total Number of Trials = 24 ÷ 40 = 0.6 P(H) + P(T) = 0.6 + 0.4 = 1 Note: Repeat this experiment for ‘n’ times and then you will find that the number of times increases, the fraction of experimental probability comes closer to 0.5. Thus if we add P(H) and P(T), we will get  0.6 + 0.4 = 1 which means P(H) and P(T) is the only possible outcomes.

Example 2. A manufacturer makes 50,000 cell phones every month. After inspecting 1000 phones, the manufacturer found that 30 phones are defective. What is the probability that you will buy a phone that is defective? Predict how many phones will be defective next month.

Experimental Probability = 30/1000 = 0.03 0.03 = (3/100) × 100 = 3% The probability that you will buy a defective phone is 3% ⇒ Number of defective phones next month = 3% × 50000 ⇒ Number of defective phones next month = 0.03 × 50000 ⇒ Number of defective phones next month = 1500

Example 3. There are about 320 million people living in the USA. Pretend that a survey of 1 million people revealed that 300,000 people think that all cars should be electric. What is the probability that someone chosen randomly does not like the electric car? How many people like electric cars?

Now the number of people who do not like electric cars is 1000000 – 300000 = 700000 Experimental Probability =  700000/1000000 = 0.7 And, 0.7 = (7/10) × 100 = 70% The probability that someone chose randomly does not like the electric car is 70% The probability that someone like electric cars is  300000/1000000 = 0.3 Let x be the number of people who love electric cars ⇒ x = 0.3 × 320 million ⇒ x = 96 million The number of people who love electric cars is 96 million.

Practice Problems on Experimental Probability

Problem 1: A coin is flipped 200 times, and it lands on heads 120 times. What is the experimental probability of getting heads?

Problem 2: A die is rolled 50 times, and the number 3 appears 8 times. What is the experimental probability of rolling a 3?

Problem 3: In a class survey, 150 students were asked if they prefer reading books or watching movies. 90 students said they prefer watching movies. What is the experimental probability that a randomly chosen student prefers watching movies?

Problem 4: A bag contains 5 red, 7 blue, and 8 green marbles. If 40 marbles are drawn at random with replacement, and 12 of them are red, what is the experimental probability of drawing a red marble?

Problem 5: A basketball player made 45 successful free throws out of 60 attempts. What is the experimental probability that the player will make a free throw?

Problem 6: During a game, a spinner is spun 80 times, landing on a specific section 20 times. What is the experimental probability of the spinner landing on that section?

FAQs on Experimental Probability

Define experimental probability..

Probability of an event based on an actual trail in physical world is called experimental probability.

How is Experimental Probability calculated?

Experimental Probability is calculated using the following formula:  P(E) = (Number of trials taken in which event A happened) / Total number of trials

Can Experimental Probability be used to predict future outcomes?

No,  experimental probability can’t be used to predict future outcomes as it only achives the theorectical value when the trails becomes infinity.

How is Experimental Probability different from Theoretical Probability?

 Theoretical probability is the probability of an event based on mathematical calculations and assumptions, whereas experimental probability is based on actual experiments or trials.

What are some Limitations of Experimental Probability?

There are some limitation of experimental probability, which are as follows: Experimental probability can be influenced by various factors, such as the sample size, the selection process, and the conditions of the experiment.  The number of trials conducted may not be sufficient to establish a reliable pattern, and the results may be subject to random variation.  Experimental probability is also limited to the specific conditions of the experiment and may not be applicable in other contexts.

Can Experimental Probability of an event be a negative number if not why?

As experimental probability is given by: P(E) = Number of trials taken in which event A happened/Total number of trials Thus, it can’t be negative as both number are count of something and counting numbers are 1, 2, 3, 4, …. and they are never negative.

What are Types of Probability?

There are two forms of calculating the probability of an event that are, Theoretical Probability Experimental Probability

Similar Reads

• Mathematics
• School Learning
• Technical Scripter
• Maths-Class-9
• Probability
• Technical Scripter 2020

Please Login to comment...

Improve your coding skills with practice.

What kind of Experience do you want to share?